Sign in
Please select an account to continue using cracku.in
↓ →
The base exchange rate of a currency X with respect to a currency Y is the number of units of currency Y which is equivalent in value to one unit of currency X. Currency exchange outlets buy currency at buying exchange rates that are lower than base exchange rates, and sell currency at selling exchange rates that are higher than base exchange rates.
A currency exchange outlet uses the local currency L to buy and sell three international currencies A, B, and C, but does not exchange one international currency directly with another. The base exchange rates of A, B and C with respect to L are in the ratio 100:120:1. The buying exchange rates of each of A, B, and C with respect to L are 5% below the corresponding base exchange rates, and their selling exchange rates are 10% above their corresponding base exchange rates. The following facts are known about the outlet on a particular day:
1. The amount of L used by the outlet to buy C equals the amount of L it received by selling C.
2. The amounts of L used by the outlet to buy A and B are in the ratio 5:3.
3. The amounts of L the outlet received from the sales of A and B are in the ratio 5:9.
4. The outlet received 88000 units of L by selling A during the day.
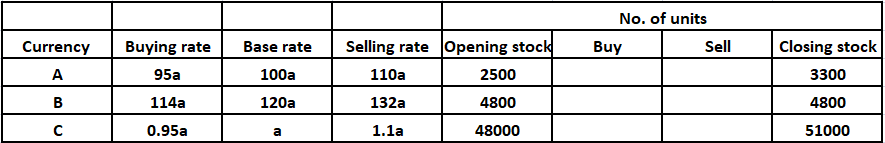
5. The outlet started the day with some amount of L, 2500 units of A, 4800 units of B, and 48000 units of C.
6. The outlet ended the day with some amount of L, 3300 units of A, 4800 units of B, and 51000 units of C.
How many units of currency A did the outlet buy on that day?
Correct Answer: 1200
It is given that the base exchange rates of A, B and C with respect to L are in the ratio 100:120:1. Let us assume that base exchange rates are '100a', '120a' and 'a' in that order.
It is given that the buying exchange rates of each of A, B, and C with respect to L are 5% below the corresponding base exchange rates. Therefore, we can say that the buying exchange rates are 95a, 114a, 0.95a.
It is given that the selling exchange rates of each of A, B, and C with respect to L are 10% above their corresponding base exchange rates. Therefore, we can say that the selling exchange rates are 110a, 132a, 1.1a.
We know about the opening and closing units in stock for each currency. Let us draw the table accordingly.
Let 'p', 'q' and 'r' be the number of units of currency A, B and C bought by the outlet on that day.
Then, we can say that the outlet sold 'p - 800', 'q' and 'r-3000' units of currency A, B and C respectively.
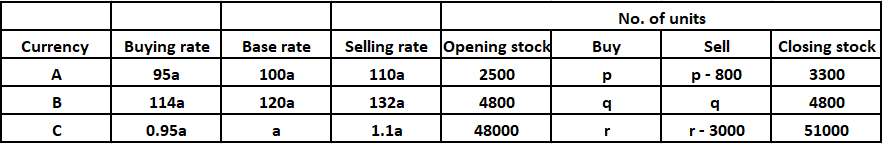
It is given that the amount of L used by the outlet to buy C equals the amount of L it received by selling C.
$$\Rightarrow$$ 0.95a*r = 1.1a*(r - 3000)
$$\Rightarrow$$ 0.15r = 3300
$$\Rightarrow$$ r = 22000
It is also given that the amounts of L used by the outlet to buy A and B are in the ratio 5:3.
$$\Rightarrow$$ $$\dfrac{p*95a}{q*114a} = \dfrac{5}{3}$$
$$\Rightarrow$$ p = 2q
Also, the amounts of L the outlet received from the sales of A and B are in the ratio 5:9.
$$\Rightarrow$$ $$\dfrac{(p-800)*110a}{q*132a} = \dfrac{5}{9}$$
$$\Rightarrow$$ $$\dfrac{(2q-800)*110a}{q*132a} = \dfrac{5}{9}$$
$$\Rightarrow$$ q = 600
Therefore, p = 2q = 2*600 = 1200.
It is given that the outlet received 88000 units of L by selling A during the day.
$$\Rightarrow$$ (p-800)*110a = 88000
$$\Rightarrow$$ (1200-800)*110a = 88000
$$\Rightarrow$$ 44000a = 88000
$$\Rightarrow$$ a = 2
We can fill the entire table and answer all the questions.
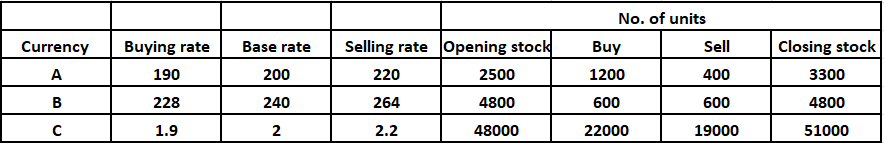
From the table we can see that the currency outlet bought 1200 units of A.

Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get: