A 10 litre cylinder contains a mixture of water and sugar, the volume of sugar being 15% of total volume. A few litres of the mixture is released and an equal amount of water is added. Then the same amount of the mixture as before is released and replaced with water for a second time. As a result, the sugar content becomes 10% of total volume. What is the approximate quantity of mixture released each time?

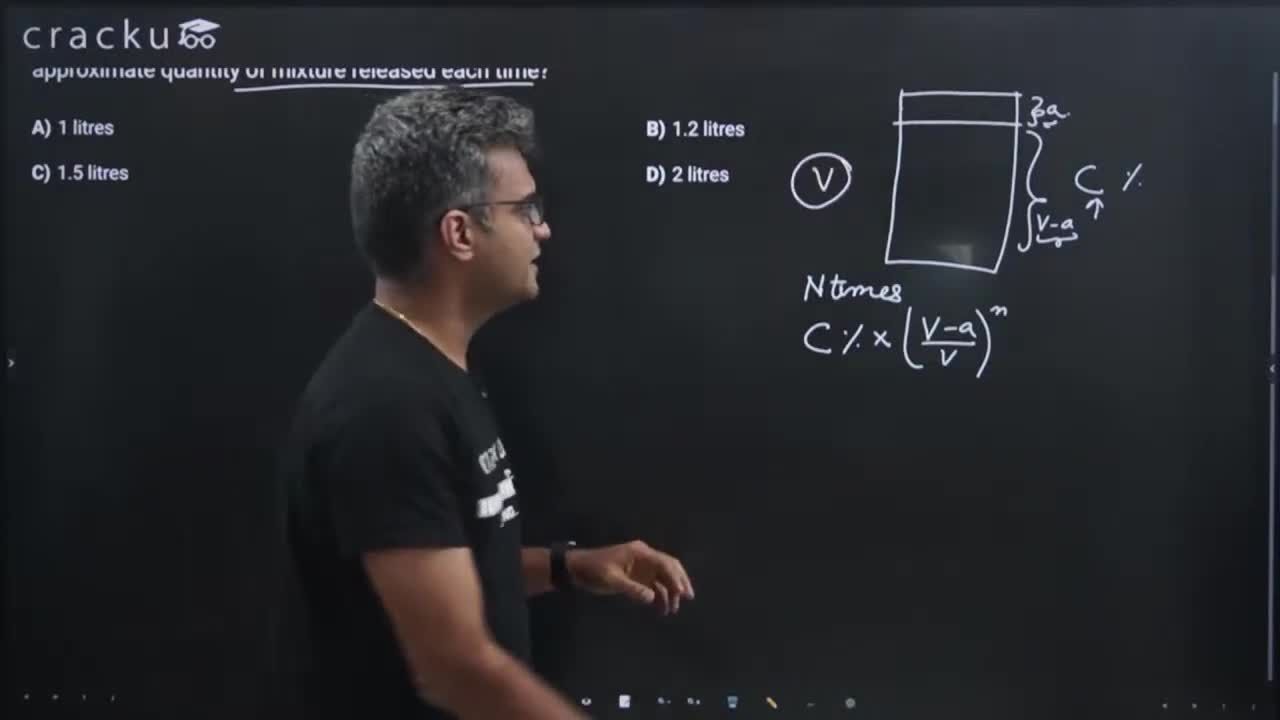
Initially in the 10L solution there is 1.5L sugar and 8.5L water.
We know that:-
Final volume of sugar = Initial volume of sugar*(Volume of solution that is not drawn out/total volume of solution)$$^n$$
 i.e $$1 = 1.5*(\dfrac{10-x}{10})^2$$ Where x is the volume of the solution drawn out.
Thus, $$200 = 3*(10^2 - 20x + x^2)$$
Solving we get x $$\approx 1.873$$
The closest value in the option is option D.
Thus, option D is the correct answer.