Given below are two statements :
Statement I: If two chords XY and ZT of a circle intersects internally at point P, then PX - PY = PZ - PT
Statement II: If two chords XY and ZT of a circle intersect internally at point P, then PXZ and PTY are similar triangles.
In the light of the above statements, choose the correct answer from the options given below:

Statement I: If two chords XY and ZT of a circle intersects internally at point P, then PX - PY = PZ - PT

There is no such theorem since the length of XY and ZT can be different. Let's take any random example:

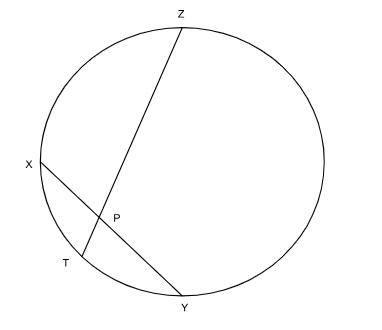
Here, we can clearly say PX - PY < PZ - PT

Hence, this statement is not true. 

Statement II: If two chords XY and ZT of a circle intersect internally at point P, then PXZ and PTY are similar triangles.

This statement is correct. When two chords intersect inside a circle, triangles formed by the intersecting chords (i.e., triangles PXZ and PTY) are indeed similar by the AA (Angle-Angle) similarity criterion. This happens because:

∠XPZ = ∠TPY (vertically opposite angles)

Now, if I join XT, the triangles formed will be XZT and XYT. Hence, the triangle formed on the same base has third angles equal. With this, we can say, Angle XZT = Angle XYT

Therefore, by the Angle-Angle (AA) similarity criterion, triangle PXZ is similar to triangle PTY.

So, the correct answer is: Statement I is false but Statement II is true.