Solution
Given, $$x+\ \frac{\ 1}{x}=2$$
$$x^2+1=2x^{ }$$
$$x^2-2x+1=0$$
$$\left(x-1\right)^2=0$$
$$x=1$$
Therefore, $$x^{100}+\ \frac{\ 1}{x^{100}}=1^{100}+\ \frac{\ 1}{1^{100}}= 1 + 1 =2$$
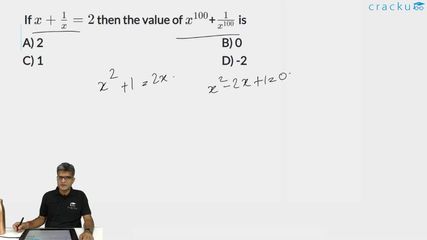
Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get:
- Free SSC Study Material - 18000 Questions
- 230+ SSC previous papers with solutions PDF
- 100+ SSC Online Tests for Free
