Two pipes can fill a tank in 12 hours and 18 hours, respectively. The pipes are opened simultaneously, and it is found that due to leakage at the bottom of the tank, it took 36 minutes more to fill the tank. When the tank is full, in what time the leak will empty it?

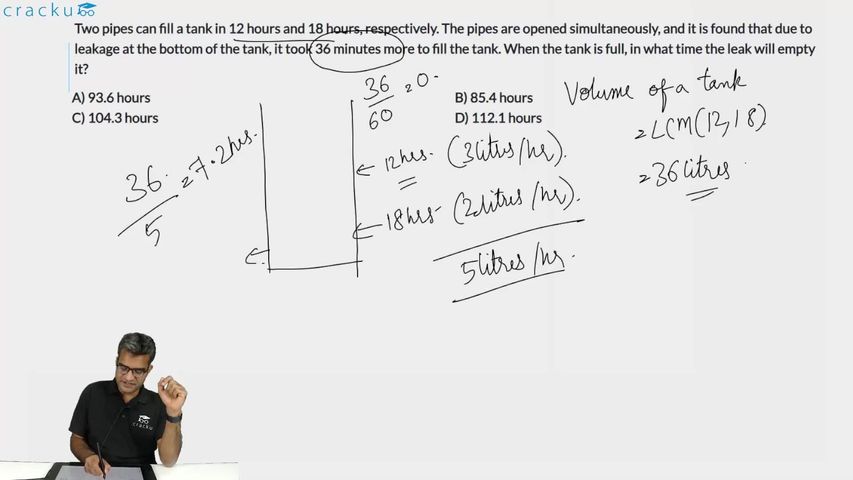
Let the volume of the tank be L.C.M of (12,18) = $$72 m^3$$

The rate of inflow of the pipes are, $$\frac{72}{12}=6\ \frac{m^3}{hr}\ \&\ \frac{72}{18}=4\ \frac{m^3}{hr}$$

Let the rate of leakage be $$x\ \frac{m^3}{hr}$$

The total time it takes for both pipes to fill the tank = $$\frac{72}{6+4}=\frac{72}{10}=7.2\ hr$$

But due to leakage, the pipes took 36 min more to fill the tank, $$\frac{72}{6+4-x}=7.2\ +\frac{36}{60}$$

$$\frac{72}{10-x}=7.8$$

on solving, we get $$x=\frac{10}{13}\ \frac{m^3}{hr}$$

The total time taken by the leak to empty the full tank = $$\frac{72}{\frac{10}{13}}=93.6\ hr$$