Please enter the following details
The game of QUIET is played between two teams. Six teams, numbered 1, 2, 3, 4, 5, and 6, play in a QUIET tournament. These teams are divided equally into two groups. In the tournament, each team plays every other team in the same group only once, and each team in the other group exactly twice. The tournament has several rounds, each of which consists of a few games. Every team plays exactly one game in each round.
The following additional facts are known about the schedule of games in the tournament.
1. Each team played against a team from the other group in Round 8.
2. In Round 4 and Round 7, the match-ups, that is the pair of teams playing against each other, were identical. In Round 5 and Round 8, the match-ups were identical.
3. Team 4 played Team 6 in both Round 1 and Round 2.
4. Team 1 played Team 5 ONLY once and that was in Round 2.
5. Team 3 played Team 4 in Round 3. Team 1 played Team 6 in Round 6.
6. In Round 8, Team 3 played Team 6, while Team 2 played Team 5.
We are told that there are six teams, that are divided into two groups.
Teams in the same group will play each other only once, and teams in different group will play each other twice.
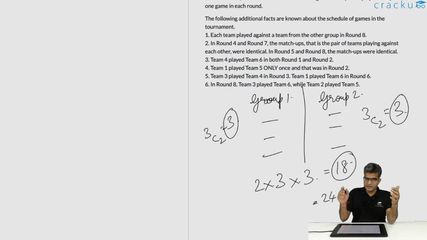
Calculating the combinations, there is going to be $$^3C_2$$ games among teams in the same group among them, and since there is two groups, total such games will be 6.
Now, teams in different group play each other twice. Calculating the combinations for this,
From the first group, a team can be chosen in three ways, and from the second group, a team can be chosen in three ways. Total ways two teams from different groups can play each other is 3x3 which is 9. And since they play each other twice, that is 9+9 games of this combination.
Total number of games is 18+6=24
It is given that each team plays one game in each round, that means there is going to be 3 matchups in each round. And given, there is 24 games to played in this format, the number of rounds will be 24/3=8
The tournament will have 8 rounds.
Create a FREE account and get:
- All Quant CAT complete Formulas and shortcuts PDF
- 38+ CAT previous year papers with video solutions PDF
- 5000+ Topic-wise Previous year CAT Solved Questions for Free
