IPM Indore 2019 Question Paper
For the following questions answer them individually
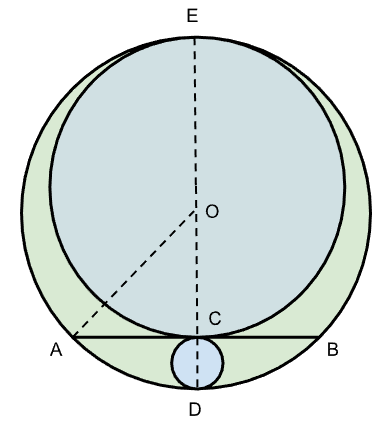
IPM Indore 2019 - Question 31
A chord is drawn inside a circle, such that the length of the chord is equal to the radius of the circle. Now, two circles are drawn, one on each side of the chord, each touching the chord at its midpoint and the original circle. Let k be the ratio of the areas of the bigger inscribed circle and the smaller inscribed circle, then k equals
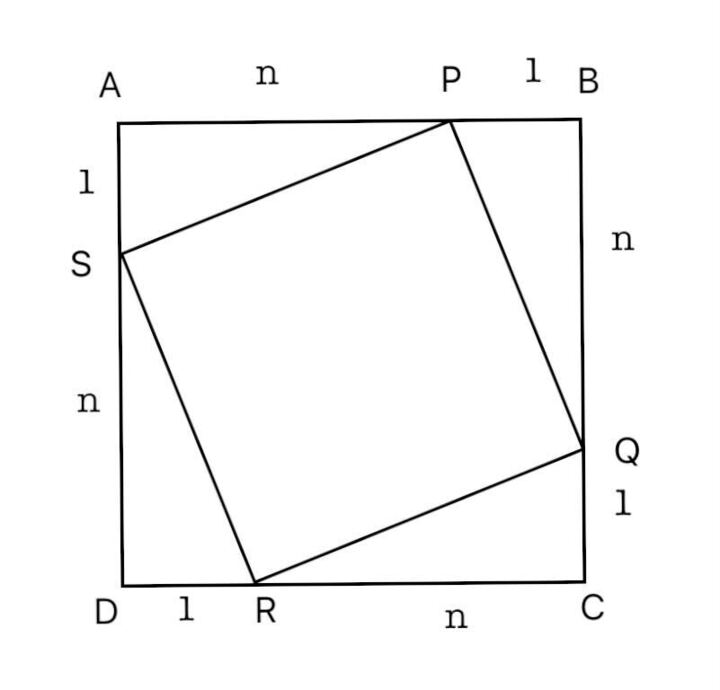
IPM Indore 2019 - Question 32
Points P, Q, R and S are taken on sides AB, BC, CD and DA of square ABCD respectively, so that AP : PB = BQ : QC = CR : RD = DS : SA = 1 : n . Then the ratio of the area of PQRS to the area of ABCD is
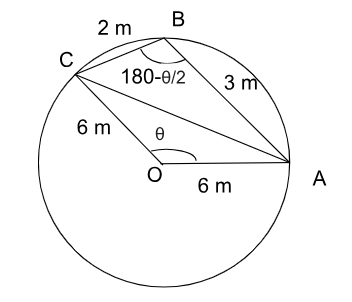
IPM Indore 2019 - Question 33
On a circular path of radius 6 m a boy starts from a point A on the circumference and walks along a chord AB of length 3 m. He then walks along another chord BC of length 2 m to reach point C. The point B lies on the minor arc AC. The distance between point C from point A is
IPM Indore 2019 - Question 35
Two points on a ground are 1 m apart. If a cow moves in the field in such a way that it's distance from the two points is always in ratio 3: 2 then
IPM Indore 2019 - Question 36
Given that $$\cos x + \cos y = 1$$, the range of $$\sin x - \sin y$$ is
IPM Indore 2019 - Question 37
If $$\sin \theta + \cos \theta = m$$, then $$\sin^{6} \theta + \cos^{6}\theta$$ equals
IPM Indore 2019 - Question 38
If inverse of the matrix $$\begin{bmatrix}2 & -0.5 \\-1 & x \end{bmatrix}$$ is $$\begin{bmatrix}1 & 1 \\2 & 4 \end{bmatrix}$$, then the value of x is
IPM Indore 2019 - Question 39
The function $$f(x) = \dfrac{x^{3} - 5x^{2} - 8x}{3}$$ is
IPM Indore 2019 - Question 40
For a > b > c > 0, the minimum value of the function f(x) = |x - a| + |x - b| + |x - c| is