Please enter the following details
Funky Pizzeria was required to supply pizzas to three different parties. The total number of pizzas it had to deliver was 800, 70% of which were to be delivered to Party 3 and the rest equally divided between Party 1 and Party 2.
Pizzas could be of Thin Crust (T) or Deep Dish (D) variety and come in either Normal Cheese (NC) or Extra Cheese (EC) versions. Hence, there are four types of pizzas: T-NC, T-EC, D-NC and D-EC. Partial information about proportions of T and NC pizzas ordered by the three parties is given below:
Suppose that a T-NC pizza cost as much as a D-NC pizza, but 3/5th of the price of a D-EC pizza.A D-EC pizza costs Rs. 50 more than a T-EC pizza, and the latter costs Rs. 500.
If 25% of the Normal Cheese pizzas delivered to Party 1 were of Deep Dish variety, what was the total bill for Party 1?
We are given that Party 3 received 70% of total pizzas,therefore, number of pizzas received by Party 3 = $$\frac{70}{100}\times 800$$ = 560
Remaining 240 pizzas are equally divided among party 1 and party 2 hence we can say that each of Party 1 and Party 2 received 120 pizzas.
We know that all of the pizza can be classified into a total of 4 types. Hence, on drawing a table which can accommodate all of the cases:
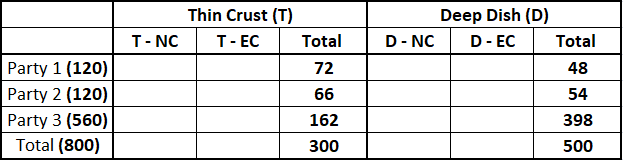
Total number of Thin Crust pizzas = 0.375*800 = 300. Therefore, total number of Deep Dish pizzas = 800 - 300 = 500.
Out of 120 pizzas that Party 1 received, 60% were of Thin Crust type hence, total number of Thin Crust pizza received by Party 1 = 0.6*120 = 72. Consequently Party 1, must have received 42 Deep Dish type pizzas.
Out of 120 pizzas that Party 2 received, 55% were of Thin Crust type hence, total number of Thin Crust pizza received by Party 2 = 0.55*120 = 66. Consequently Party 1, must have received 54 Deep Dish type pizzas.
Therefore, total number of Thin Crust pizzas ordered by Party 3 = Total Thin Crust pizzas ordered - Thin Crust pizzas ordered by Party 1 - Thin Crust pizzas ordered by Party 2
$$\Rightarrow$$ 300 - 72 - 66 = 162
Hence number of Deep Dish type of pizzas order by Party 3 = 560 - 162 = 398
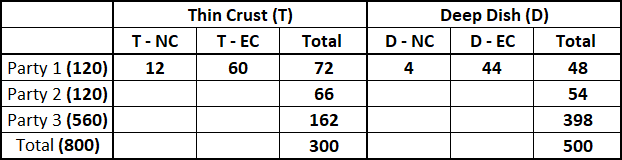
Total number of Normal Cheese pizzas require to be delivered = 0.52*800 = 416
Number of Normal Cheese pizzas require to be delivered to Party 2 = 0.3*120 = 36
Number of Normal Cheese pizzas require to be delivered to Party 3 = 0.65*560 = 364
Therefore, total number of Normal Cheese pizzas require to be delivered to Party 1 = Total Normal Cheese pizzas to be delivered - Normal Cheese pizzas require to be delivered to Party 2 - Normal Cheese pizzas require to be delivered to Party 3
\Rightarrow 416 - 36 - 364 = 16
It is given that 25% of these 16 Normal Cheese pizzas were of Deep Dish type, hence the number of D- NC type pizza require to be delivered to Party 1 = 0.25*16 = 4
Consequently, the number of T- NC type pizza require to be delivered to Party 1 = 16 - 4 = 12
We can find out each type of pizza that is required to be delivered to Party 1.
Cost Price of a T-EC pizza = Rs. 500
Cost Price of a D-EC pizza = Rs. 550
Cost Price of a T-NC pizza = $$\frac{3}{5}\times 550$$ = Rs. 330
Cost Price of a D-NC pizza = $$\frac{3}{5}\times 550$$ = Rs. 330
Therefore the total bill amount for Party 1 = 12*330 + 60*500 + 4*330 + 44*550 = Rs. 59480
Therefore, option A is the correct answer.
Video Solution
Create a FREE account and get:
- All Quant CAT complete Formulas and shortcuts PDF
- 38+ CAT previous year papers with video solutions PDF
- 5000+ Topic-wise Previous year CAT Solved Questions for Free
