Sign in
Please select an account to continue using cracku.in
↓ →
The base of a regular pyramid is a square and each of the other four sides is an equilateral triangle, length of each side being 20 cm. The vertical height of the pyramid, in cm, is
It is given that the base of the pyramid is square and each of the four sides are equilateral triangles.
Length of each side of the equilateral triangle = 20cm
Since the side of the triangle will be common to the square as well, the side of the square = 20cm
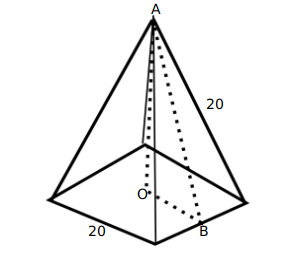
Let h be the vertical height of the pyramid ie OA
OB = 10 since it is half the side of the square
AB is the height of the equilateral triangle i.e 10$$\sqrt{\ 3}$$
AOB is a right angle, so applying the Pythagorean formula, we get
$$OA^2+ OB^2= AB^2$$
$$h^2$$ + 100= 300
h=10$$\sqrt{\ 2}$$

Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get: