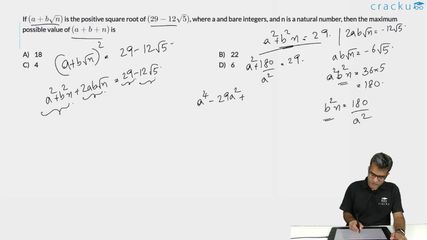
If $$(a + b \sqrt{n})$$ is the positive square root of $$(29 - 12\sqrt{5})$$, where a and b are integers, and n is a natural number, then the maximum possible value of $$(a + b + n)$$ is

$$(a + b \sqrt{n})$$ is the positive square root of $$(29 - 12\sqrt{5})$$

So $$29-12\sqrt{5}=\left(a+b\sqrt{n}\right)^2$$
$$29-12\sqrt{5}=a^2+b^2n+2ab\sqrt{n}$$

$$a^2+b^2n=29$$ and
$$ab\sqrt{n}=-6\sqrt{5}$$
$$a^2b^2n=180$$
$$b^2n=\frac{180}{a^2}$$
Substituting this in the above equation, 
$$a^2+\frac{180}{a^2}=29$$
$$a^4-29a^2+180=0$$
$$a^2=\frac{\left(29\pm\sqrt{29^2-4\left(180\right)}\right)}{2}$$
$$a^2=\frac{\left(29+\sqrt{841-720}\right)}{2}$$
$$a^2=9\ or\ 20$$

That means, one of $$a^2\ or\ b^2n$$ is 9 and 20. 

We also have, $$ab\sqrt{n}=-6\sqrt{5}$$ that means one of a or b should be negative
And also the fact that this is a positive square root,
And we need to maximise the value of a, b and n. 

We can have a=-3, b=1 and n=20. 
This satisfies all the above equations, and the value of a+b+n=18.