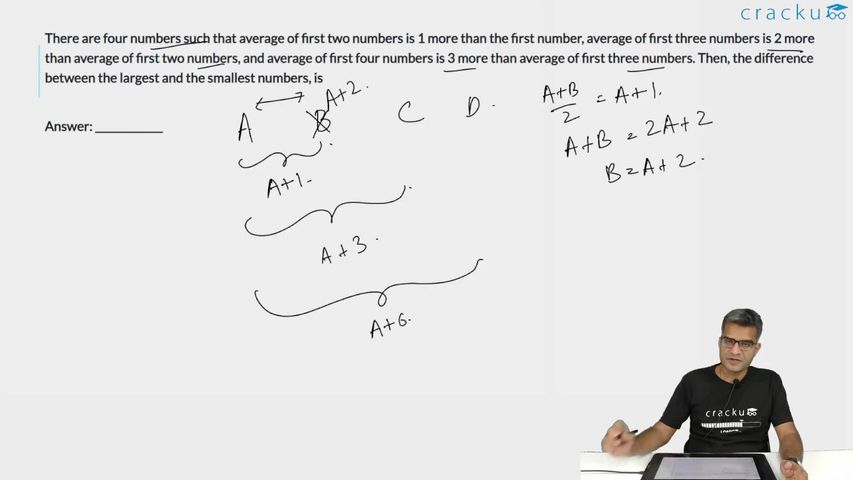
There are four numbers such that average of first two numbers is 1 more than the first number, average of first three numbers is 2 more than average of first two numbers, and average of first four numbers is 3 more than average of first three numbers. Then, the difference between the largest and the smallest numbers, is

Let us assume the four numbers to be a, b, c and d in ascending order. 

Average of first two numbers is 1 more than the first number
$$\frac{\left(a+b\right)}{2}=a+1$$
$$b-a=2$$
$$b=a+2$$

Average of first three numbers is 2 more than average of first two numbers
$$\frac{\left(a+b+c\right)}{3}=\frac{\left(a+b\right)}{2}+2$$
$$2c=a+b+12$$
Substituting the value for b
$$2c=a+a+2+12$$
$$2c=2a+14$$
$$c=a+7$$

Average of first four numbers is 3 more than average of first three numbers.
$$\frac{\left(a+b+c+d\right)}{4}=\frac{\left(a+b+c\right)}{3}+3$$
$$3d=a+b+c+36$$
Substituting the value of b and c
$$3d=a+a+2+a+7+36$$
$$3d=3a+45$$
$$d=a+15$$

d is the largest and a is the smallest and we know that d=a+15

Hence the difference between the smallest and the largest values is 15.