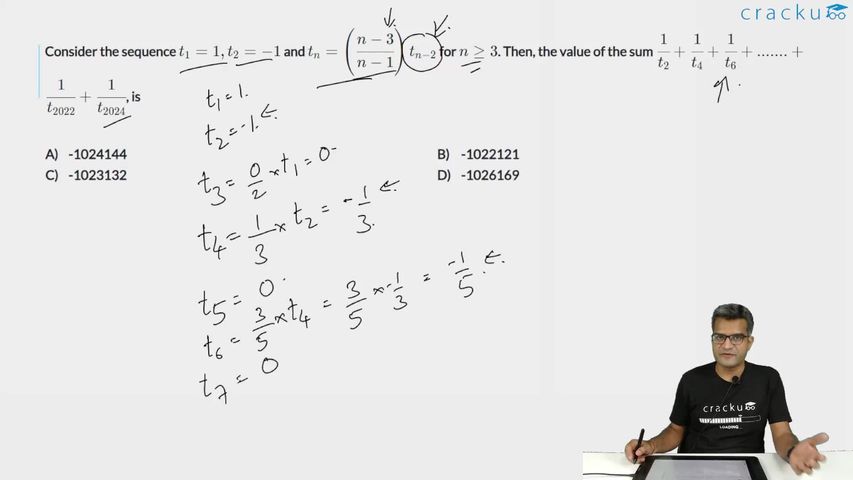
Consider the sequence $$t_1 = 1, t_2 = -1$$ and $$t_n = \left(\cfrac{n - 3}{n - 1}\right)t_{n - 2}$$ for $$n \geq 3$$. Then, the value of the sum $$\cfrac{1}{t_2} + \cfrac{1}{t_4} + \cfrac{1}{t_6} + ....... +\cfrac{1}{t_{2022}} + \cfrac{1}{t_{2024}}$$, is

Finding the terms in the sequence, we see that $$t_3=0$$, $$t_4=-\frac{1}{3}$$, $$t_5=0$$
We would notice that all the odd terms are 0, and we are also asked the sum of only even terms, so we do not need to consider those

$$t_6=-\frac{1}{5}$$
We see that the even terms are in an HP: $$-1,\ -\frac{1}{3},\ -\frac{1}{5},\ -\frac{1}{7},\ ...$$

The sum we are asked is the inverse of these terms, that is: -1, -3, -5, -7, up to 1012 terms

The sum of this AP would be $$\frac{\left[-\left(2\times\ 1\right)+\left(1012-1\right)\left(-2\right)\right]}{2}\times\ 1012$$
Which is equal to $$-1012\times\ 1012\ =\ -1024144$$

Therefore, Option A is the correct answer.