Fun Sports (FS) provides training in three sports - Gilli-danda (G), Kho-Kho (K), and Ludo (L). Currently it has an enrollment of 39 students each of whom is enrolled in at least one of the three sports. The following details are known:
1. The number of students enrolled only in L is double the number of students enrolled in all the three sports.
2. There are a total of 17 students enrolled in G.
3. The number of students enrolled only in G is one less than the number of students enrolled only in L.
4. The number of students enrolled only in K is equal to the number of students who are enrolled in both K and L.
5. The maximum student enrollment is in L.
6. Ten students enrolled in G are also enrolled in at least one more sport.
What is the minimum number of students enrolled in both G and L but not in K?
Correct Answer: 4
Let 'x' be the number of students enrolled in all three sports. Then the number ofĀ students enrolled only in L = 2x
It is given thatĀ there are a total of 17 students enrolled in G. Also, ten students enrolled in G are also enrolled in at least one more sport. Hence, the number of students enrolled in only G = 17 - 10 = 7
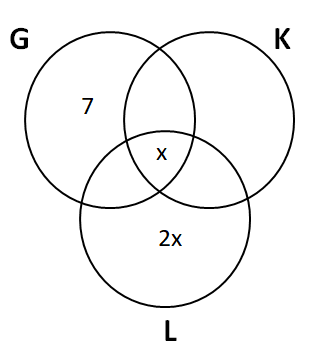
The number of students enrolled only in G is one less than the number of students enrolled only in L. Hence, the number of students enrolled only in L = 7+1Ā
$$\Rightarrow$$ 2x = 8Ā
$$\Rightarrow$$ x = 4
Let us assume that 'y' students are enrolled in K and L but not G. Then, the number of studentsĀ enrolled only in K = y + 4
Let us assume that 'z' be the the number of students enrolled in G and K but not L. Then, theĀ number of studentsĀ enrolled G and L bot not K = 10 - 4 - z = 6 - z
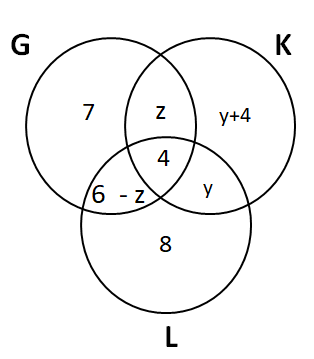
It is given that a total of 39 students in the sports.Ā
7 + z + 4 + 6 - z + 8 + y + y + 4 = 39Ā
$$\Rightarrow$$ y = 5Ā
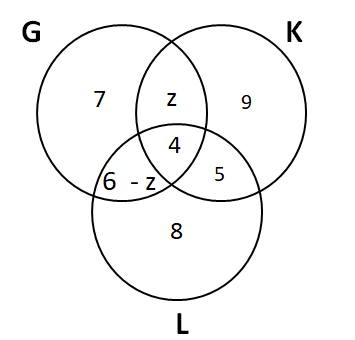
Number of students enrolled in G = 17Ā
Number of students enrolled in K = 9 + 4 + 5 + z = 18 + z
Number of students enrolled in L = 6 - z + 4 + 5 + 8 = 23 - z
It is given thatĀ the maximum student enrollment is in L.
$$\Rightarrow$$ 23 - z > 18 + z
$$\Rightarrow$$ 2z < 5
$$\Rightarrow$$ z < 2.5Ā
Therefore, we can say that z can take three values = {0, 1, 2}
The number of studentsĀ Ā enrolled in both G and L but not in K = 6 - z. This number will be minimum when 'z' is maximum. z_{max} = 2
Therefore,Ā the minimum number of students enrolled in both G and L but not in K = 6 - 2 = 4
Video Solution
Create a FREE account and get:
- All Quant CAT complete Formulas and shortcuts PDF
- 35+ CAT previous papers with video solutions PDF
- 5000+ Topic-wise Previous year CAT Solved Questions for Free
