Sign in
Please select an account to continue using cracku.in
↓ →
In a circle, two parallel chords on the same side of a diameter have lengths 4 cm and 6 cm. If the distance between these chords is 1 cm, then the radius of the circle, in cm, is
Given that two parallel chords on the same side of a diameter have lengths 4 cm and 6 cm.
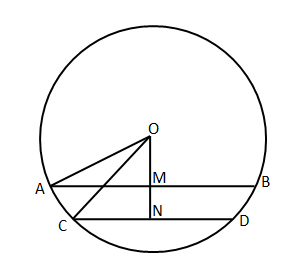
In the diagram we can see that AB = 6 cm, CD = 4 cm and MN = 1 cm.
We can see that M and N are the mid points of AB and CD respectively.
AM = 3 cm and CD = 2 cm. Let 'OM' be x cm.
In right angle triangle AMO,
$$AO^2 = AM^2 + OM^2$$
$$\Rightarrow$$ $$AO^2 = 3^2 + x^2$$ ... (1)
In right angle triangle CNO,
$$CO^2 = CN^2 + ON^2$$
$$\Rightarrow$$ $$CO^2 = 2^2 + (OM+MN)^2$$
$$\Rightarrow$$ $$CO^2 = 2^2 + (x+1)^2$$ ... (2)
We know that both AO and CO are the radius of the circle. Hence $$AO = CO$$
Therefore, we can equate equation (1) and (2)
$$3^2+x^2$$=$$2^2+(x+1)^2$$
$$\Rightarrow$$ x = 2 cm
Therefore, the radius of the circle
$$AO = \sqrt{AM^2 + OM^2}$$
$$\Rightarrow$$ $$AO=\sqrt{3^2+2^2}=\sqrt{13}$$. Hence, option A is the correct answer.

Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get: