IPM Indore 2023 Question Paper

If the harmonic mean of the roots of the equation $$(5 + \sqrt{2})x^{2} − bx + 8 + 2\sqrt{5} = 0$$ is 4 then the value of b is

Let [x] denote the greatest integer not exceeding x and {x} = x -[x].
If n is a natural number, then the sum of all values of x satisfying the equation 2[x] = x + n{x} is

If $$\frac{a+b}{b+c} = \frac{c+d}{d+a}$$, which of the following statements is always true?

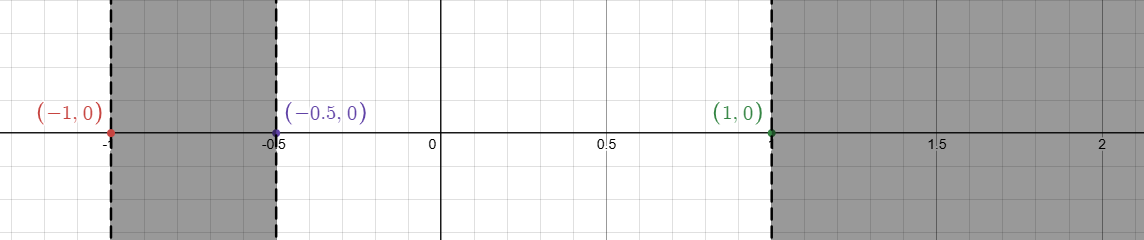
The set of all real values of x satisfying the inequality $$\frac{x^{2}(x + 1)}{(x - 1)(2x + 1)^{3}}> 0$$ is

In a chess tournament there are 5 contestants. Each player plays against all the others exactly once. No game results in a draw. The winner in a game gets one point and the loser gets zero point. Which of the following sequences cannot represent the scores of the five players?

If $$\cos \alpha + \cos \beta = 1$$ ,then the maximum value of $$\sin \alpha − \sin \beta$$ is

A polynomial P(x) leaves a remainder 2 when divided by (x - 1) and a remainder 1 when divided by (x-2). The remainder when P(x) is divided by (x - 1) (x - 2) is

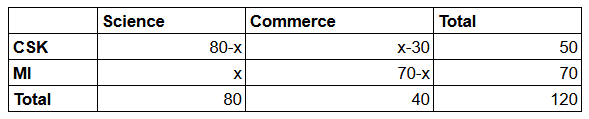
In a group of 120 students, 80 students are from the Science stream and the rest are from the Commerce stream. It is known that 70 students support Mumbai Indians in the Indian Premier League; all the other students support Chennai Super Kings. The number of Science students who are supporters of Mumbai Indians is

Let p be a positive integer such that the unit digit of $$p^{3}$$ is 4. What are the possible unit digits of $$(p+3)^{3}$$?

If a three-digit number is chosen at random, what is the probability that it is divisible neither by 3 nor by 4?