IPM Indore 2023 Question Paper

The probability that a randomly chosen positive divisor of $$10^{2023}$$ is an integer multiple of $$10^{2001}$$ is

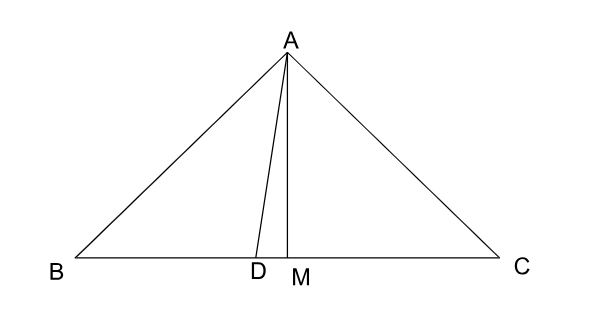
In a triangle ABC, let D be the mid-point of BC, and AM be the altitude on BC. If the lengths of AB, BC and CA are in the ratio of 2:4:3, then the ratio of the lengths of BM and AD would be

If $$A = \begin{bmatrix}1 & 2 \\3 & a \end{bmatrix}$$ where as is a real number and det $$(A^{3} − 3A^{2} − 5A) =0$$ then one of the value of a can be

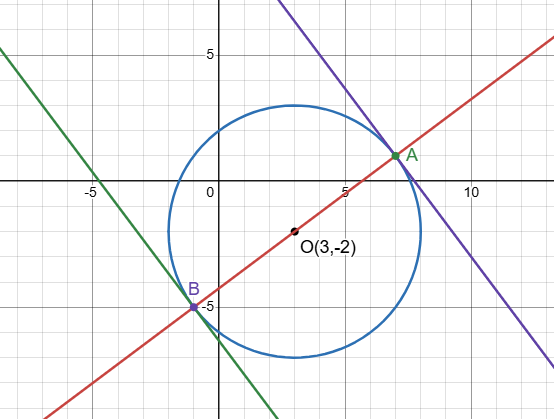
Which of the following straight lines are both tangent to the circle $$x^{2} + y^{2} − 6x + 4y − 12 = 0$$

Let $$a_{1} a_{2}, a_{2}$$ be three distinct real numbers in geometric progression. If the equations $$a_{1}x^{2} + 2a_{2} x + a_{3} = 0$$ and $$b_{1}x^{2} + 2b_{2}x + b_{3} = 0$$ has a common root,then which of the following is necessarily true?

A rabbit is sitting at the base of a staircase which has 10 steps. It proceeds to the top of the staircase by climbing either one step at a time or two steps at a time. The number of ways it can reach the top is

The minimum number of times a fair coin must be tossed so that the probability of getting at least one head exceeds 0.8 is

Consider an 8 $$\times$$ 8 chessboard. The number of ways 8 rooks can be placed on the board such that no two rooks are in the same row and no two are in the same column is

Let a, b, c be real numbers greater than 1, and n be a positive real number not equal to 1. If $$\log_{n}(\log_{2}a) = 1, \log_{n} (log_{2}b) = 2$$ and $$\log_{n}(\log_{2}c) = 3$$, then which of the following is true?

If the difference between compound interest and simple interest for a certain amount of money invested for 3 years at an annual interest rate of 10% is INR 527, then the amount invested in INR is