IPM Indore 2021 Question Paper

Suppose that a real-valued function f(x) of real numbers satisfies f(x + xy) = f(x) + f(xy) for all real x, y, and that f(2020) = 1. Compute f(2021)

Suppose that $$\log_{2} [\log_{3}(\log_{4}a)] = \log_{3} [\log_{4} (\log_{2}b)] = \log_{4} [\log_{2} (\log_{3}c)] = 0$$. Then the value of a + b + c is

Let $$S_{n}$$ be sum of the first n terms of an A.P. {$$a_{n}$$}. If $$S_{5} = S_{9}$$, what is the ratio of $$a_{3} = a_{5}$$

If A, B and A + B are non-singular matrices and AB = BA, then $$2A — B — A(A + B)^{−1}A + B(A + B)^{−1}B$$ equals

If the angles A, B,C of a triangle are in arithmetic progression such that $$\sin(2A + B) = 1/2$$ then $$\sin(B + 2C)$$ is equal to

The unit digit in $$(743)^{85} −(525)^{37} + (987)^{96}$$ IS ________

The set of all real values of p for which the equation $$3 \sin^{2}x + 12 \cos x - 3 = p$$ has at least one solution is

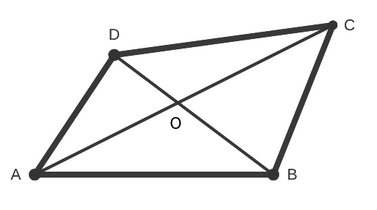
ABCD is a quadrilateral whose diagonals AC and BD intersect at O. If triangles AOB and COD have areas 4 and 9 respectively, then the minimum area that ABCD can have is

The highest possible value of the ratio of a four digit number and the sum of its four digits is:

Consider the polynomials/$$(x) = ax^{2} + bx + c$$, where a > 0, b, c are real, and g(x) = -2x. If f(x) cuts x-axis at (- 2,0) and g(x) passes through (a, b), then the minimum value of f(x) + 9 a + 1 is