IPM Indore 2021 Question Paper
For the following questions answer them individually
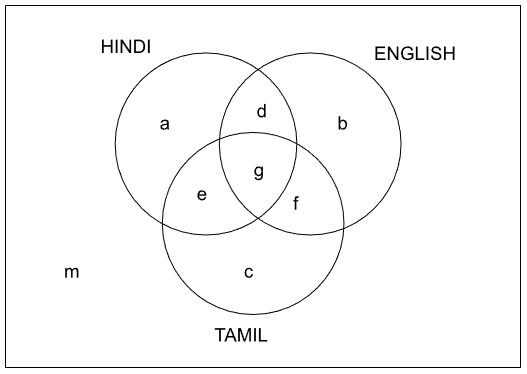
IPM Indore 2021 - Question 21
In a city, 50% of the population can speak in exactly one language among Hindi, English and Tamil, while 40% of the population can speak in at least two of these three languages. Moreover, the number of people who cannot speak in any of these three languages is twice the number of people who can speak in all these three languages. If 52% of the population can speak in Hindi and 25% of the population can speak exactly in one language among English and Tamil, then the percentage of the population who can speak in Hindi and in exactly one more language among English and Tamil is _______________.
IPM Indore 2021 - Question 22
A train left point A at 12 noon. Two hours later, another train started from point A in the same direction. It overtook the first train at 8 PM. It is known that the sum of the speeds of the two trains is 140 km/hr. Then, at what time would the second train overtake the first train, if instead the second train had started from point A in the same direction 5 hours after the first train? Assume that both the trains travel at constant speeds.
IPM Indore 2021 - Question 23
The number of 5-digit numbers consisting of distinct digits that can be formed such that only odd digits occur at odd places is
IPM Indore 2021 - Question 24
There are 10 points in the plane, of which 5 points are collinear and no three among the remaining are collinear. Then the number of distinct straight lines that can be formed out of these 10 points is
IPM Indore 2021 - Question 25
The x-intercept of the line that passes through the intersection of the lines x + 2y = 4 and 2x + 3y = 6, and is perpendicular to the line 3x — y = 2 is
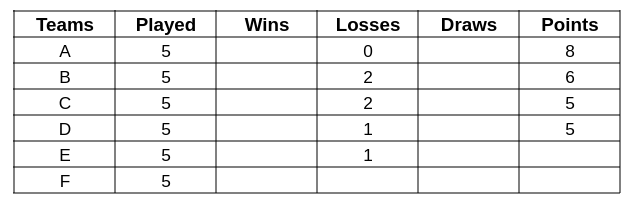
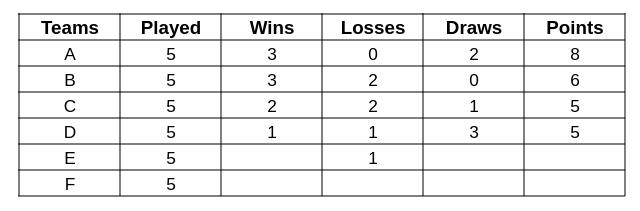
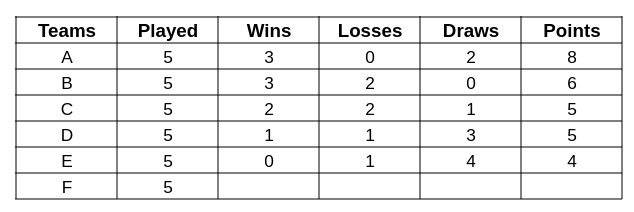
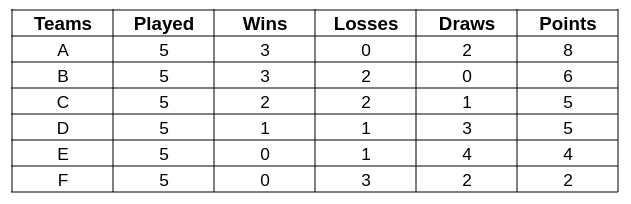
In a football tournament six teams A, B, C, D, E and F participated. Every pair of teams had exactly one match among them. For any team, a win fetches 2 points, a draw fetches 1 point, and a loss fetches no points. Both the teams E and F ended with less than 5 points. At the end of the tournament points table is as follows (some of the entries are not shown):

It is known that: (1) Team B defeated Team C, and (2) Team C defeated Team D.