Sign in
Please select an account to continue using cracku.in
↓ →
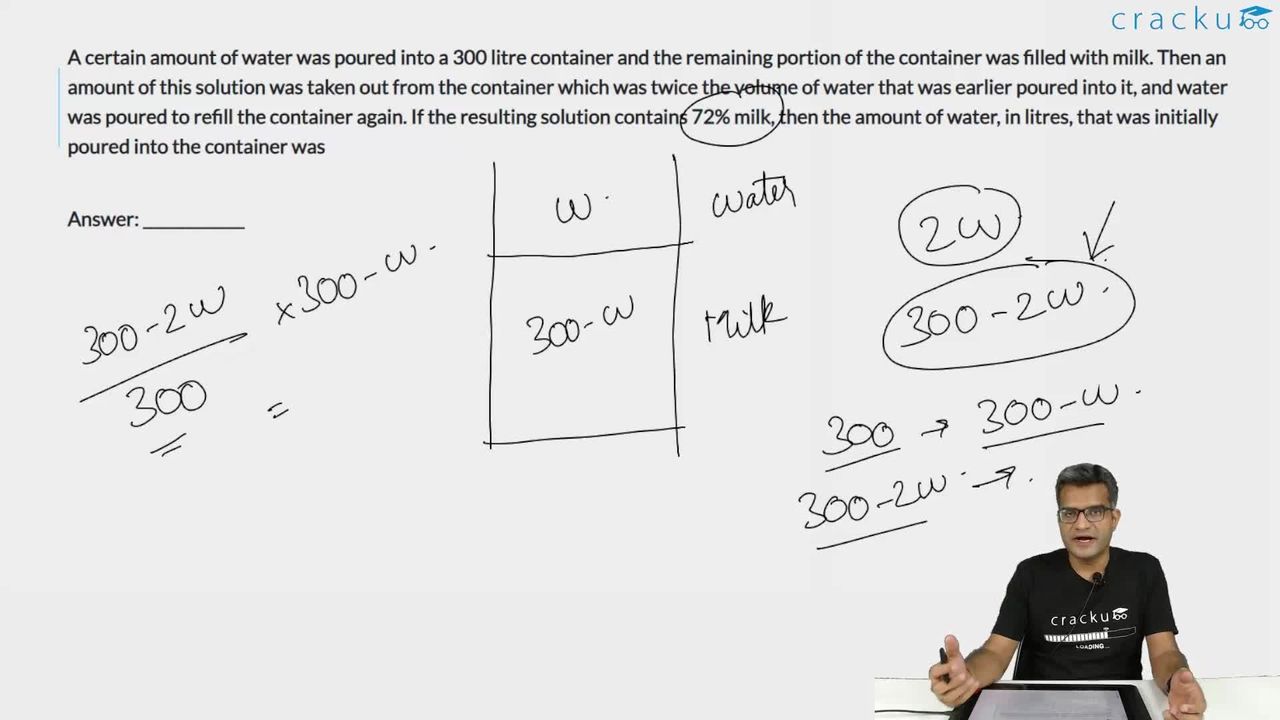
A certain amount of water was poured into a 300 litre container and the remaining portion of the container was filled with milk. Then an amount of this solution was taken out from the container which was twice the volume of water that was earlier poured into it, and water was poured to refill the container again. If the resulting solution contains 72% milk, then the amount of water, in litres, that was initially poured into the container was
Correct Answer: 30
Let us assume the amount of Milk in the container to be X and the amount of water in the container to be Y.
We are told that X+Y=300.
Now, an operation is given where "an amount of this solution was taken out from the container which was twice the volume of water that was earlier poured into it, and water was poured to refill the container again"
Volume of the water initially is Y. If twice that amount is taken out, the percentage of the solution that is taken out will be, $$\frac{2Y}{X+Y}$$
That means the quantity of milk that will remain in the solution will be, $$X\left(1-\frac{2Y}{X+Y}\right)$$
This value is given to be 72%, 72% of 300 will be 216
$$X\left(1-\frac{2Y}{X+Y}\right)=216$$
$$X\left(\frac{X+Y-2Y}{X+Y}\right)=216$$
Writing $$X=300-Y$$
$$\left(300-Y\right)\left(300-2Y\right)=64800$$
Expanding this we have,
$$2Y^2-900Y+25200=0$$
Factorising this equation we have,
$$2\left(Y-30\right)\left(Y-420\right)=0$$
Y is either 30 or 420.
Given that the capacity of the container itself is 300, Y has to be 30.
Hence the amount of water initially is 30 Litres.
Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get: