IPM Indore 2020 Question Paper

The probability that a randomly chosen factor of $$10^{19}$$ is a multiple of $$10^{15}$$ is

The number of acute angled triangles whose sides are three consecutive positive integers and whose perimeter is at most 100 is

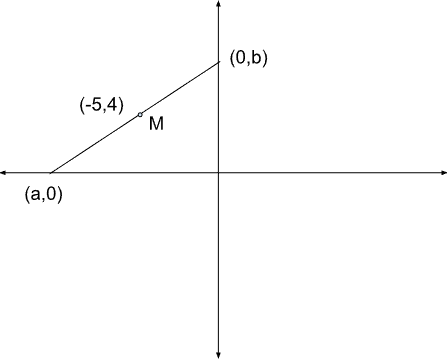
The equation of the straight line passing through the point M (-5,4), such that the portion of it between the axes is divided by the point M is to two equal halves, is

The value of $$\cos^{2}\frac{\pi}{8} + \cos^{2}\frac{3\pi}{8} + \cos^{2}\frac{5\pi}{8} + \cos^{2}\frac{7\pi}{8} + $$ is

If $$\frac{1}{1^{2}} + \frac{1}{2^{2}} + \frac{1}{3^{2}} + $$...... upto $$ \infty = \frac{\pi^{2}}{6}$$, then value of $$\frac{1}{1^{2}} + \frac{1}{3^{2}} + \frac{1}{5^{2}} + $$...... upto $$\infty$$ is

A man is known to speak the truth on an average 4 out of 5 times. He throws a die and reports that it is a five. The probability that it is actually a five is

If $$\log_{5}\log_{8}(x^2 - 1) = 0$$, then a possible value of x is

Consider the following statements:
(a) When 0 < x < 1, then $$\frac{1}{1+x} < 1 - x + x^{2}$$.
(b) When 0 < x < 1, then $$\frac{1}{1+x} > 1 - x + x^{2}$$.
(c) When -1 < x < 0, then $$\frac{1}{1+x} < 1 - x + x^{2}$$.
(d) When -1 < x < 0, then $$\frac{1}{1+x} > 1 - x + x^{2}$$.

Fifty litres of a mixture of milk and water contains 30 percent of water. This mixture is added to eighty litres of another mixture of milk and water that contains 20 percent of water. Then, how many litres of water should be added to the resulting mixture to obtain a final mixture that contains 25 percent of water?

Three workers working together need 1 hour to construct a wall. The first worker, working alone, can construct the wall twice as fast at the third worker, and can complete the task an hour sooner than the second worker. Then, the average time in hours taken by the three workers, when working along, to construct the wall is