IPM Indore 2019 Question Paper

The sum of the interior angles of a convex n-sided polygon is less than $$2019^{\circ}$$. The maximum possible value of n is

Suppose that a, b, and c are real numbers greater than 1. Then the value of $$\frac{1}{1+\log a^{2} c\frac{c}{a}} + \frac{1}{1+\log b^{2} c\frac{a}{b}} + \frac{1}{1+\log c^{2} a\frac{b}{c}}$$ is .............

A real-valued function f satisfies the relation f(x)f(y) = f(2xy + 3) + 3f(x + y) - 3f(y) + 6y, for all real numbers x and y, then the value of f(8) is .............

Let A, B, C be three 4 4 matrices such that det A = 5, det B = -3, and det $$C = \frac{1}{2}$$. Then the det $$(2AB^{-1}C^{3}B^{T})$$ is ........

If A is a 3 X 3 non-zero matrix such that $$A^{2} = 0$$ then determinant of $$[(1 + A)^{2}- 50A]$$ is equal to

Three friends divided some apples in the ratio 3 : 5 : 7 among themselves. After consuming 16 apples they found that the remaining number of apples with them was equal to largest number of apples received by one of them at the beginning. Total number of apples these friends initially had was

A shopkeeper reduces the price of a pen by 25% as a result of which the sales quantity increased by 20%. If the revenue made by the shopkeeper decreases by x%

For all real values of x, $$\frac{3x^{2} - 6x + 12}{x^{2} + 2x + 4}$$ lies between 1 and k, and does not take any value above k. Then k equals...........

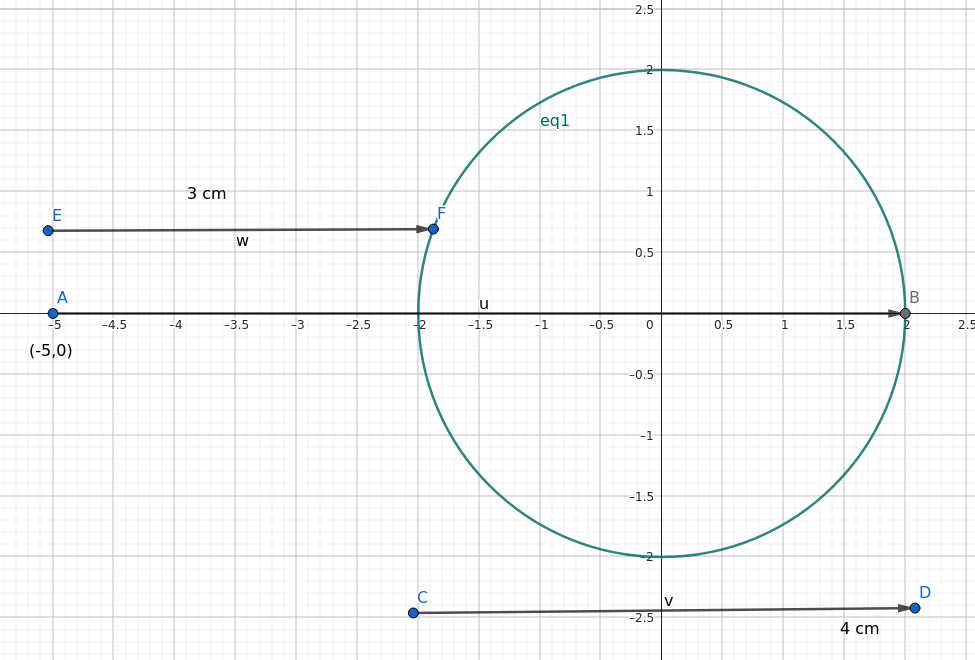
The maximum distance between the point (-5, 0) and a point on the circle $$X^{2} + Y^{2} = 4$$ is .......

If x, y, z are positive real numbers such that $$x^{12} = y^{16} = z^{24}$$, and the three quantities $$3\log_{y}x, 4 \log_{z}y, n\log_{x}z$$ are in arithmetic progression, then the value of n is