A vertical tower OP stands at the center O of a square ABCD. Let h and b denote the length OP and AB respectively. Suppose $$\angle{APB}$$ = 60° then the relationship between h and b can be expressed as

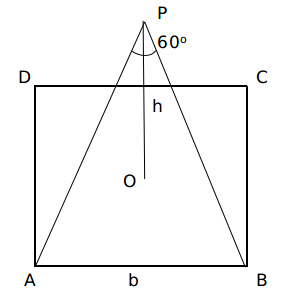
Consider the triangle APB.  $$\angle$$P = 60 and AP = BP => APB is an equilateral triangle.

Hence AP = $$b$$    ...(1)

$$\text{AC}^2 = \text{AB}^2 + \text{BC}^2$$

$$\text{AC}^2 = b^2 + b^2$$  =>  $$\text{AC} = \sqrt{2}\times b$$  =>  $$\text{AO} = \dfrac{\text{AC}}{2} = \dfrac{b}{\sqrt{2}}$$

$$\text{AP}^2 = \text{AO}^2 + \text{OP}^2$$

$$b^2 = \dfrac{b^2}{2} + h^2$$    ...From (1)

$$2h^2 = b^2$$

Hence, option B is the correct answer.