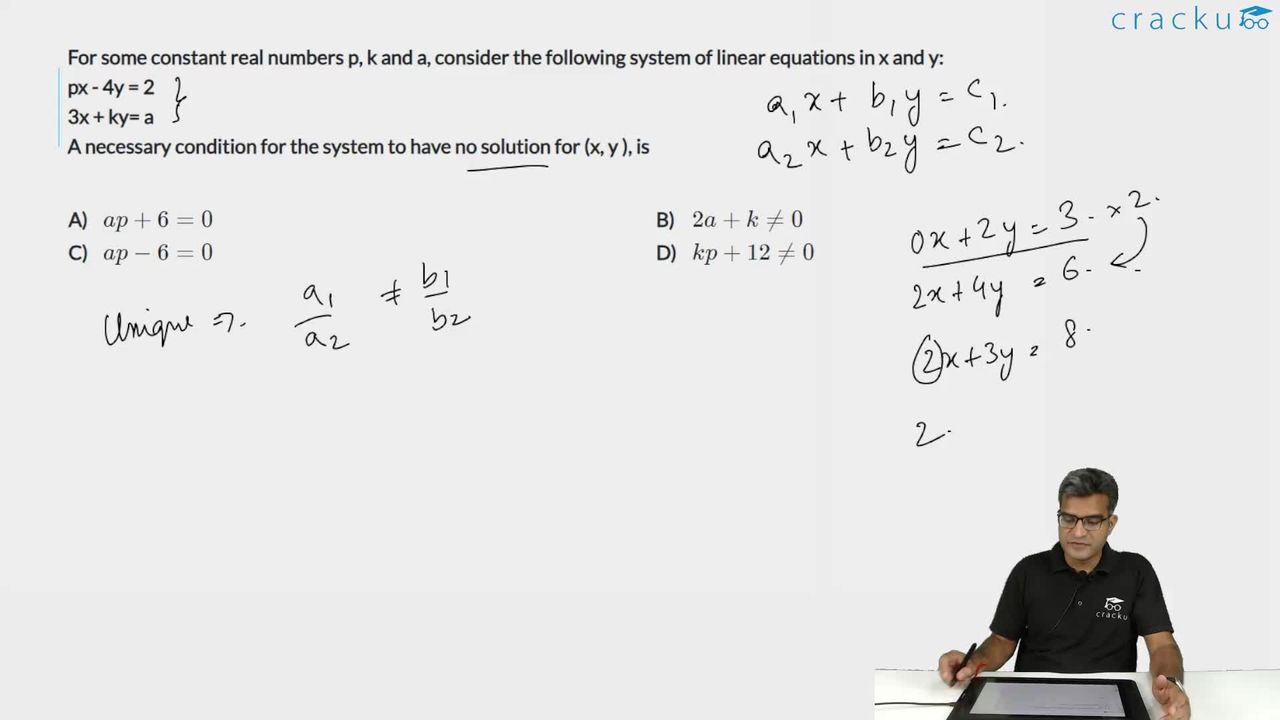
For some constant real numbers p, k and a, consider the following system of linear equations in x and y:
px - 4y = 2
3x + ky= a
A necessary condition for the system to have no solution for (x, y ), is

Arranging the equation, we know that there are no solutions when the lines are parallel:
for that the condition had to $$\frac{p}{3}=-\frac{4}{k}\ne\ \frac{2}{a}$$

Checking through options: 
Option A: using the first and last terms in our relation, we see that ap must not be equal to 6 for the lines to be parallel. This option puts no conditions on that and thus is not relevant. 

Option C: This question is the opposite of what we want; if this is true, the lines can never be parallel. 

Option D: Using the first and second terms of the relation, we see that we want kp = -12, or kp-12 = 0. Hence, this statement is not what we want. 

Option B: Using the second and third terms, we see that we do not want k equals to -2a, or we do not want k+2a to be equal to 0

Therefore, B is a condition that is necessary for the lines to be parallel and have no solution. 

Therefore, Option B is the correct answer.