In the XY-plane, the area, in sq. units, of the region defined by the inequalities
$$y \geq x + 4$$ and $$-4 \leq x^2 + y^2 + 4(x - y) \leq 0$$ is

We have two inequalities,

$$y\ \ge\ x\ +\ 4$$

$$-4\ \le\ x^2\ +\ y^2\ +\ 4\left(x\ -\ y\right)\ \le\ 0$$

The second inequality can be written as two separate inequalities,

$$-4\ \le\ x^2\ +\ y^2\ +\ 4\left(x\ -\ y\right)$$ and $$\ x^2\ +\ y^2\ +\ 4\left(x\ -\ y\right)\ \le\ 0$$

The first inequality can be written as,

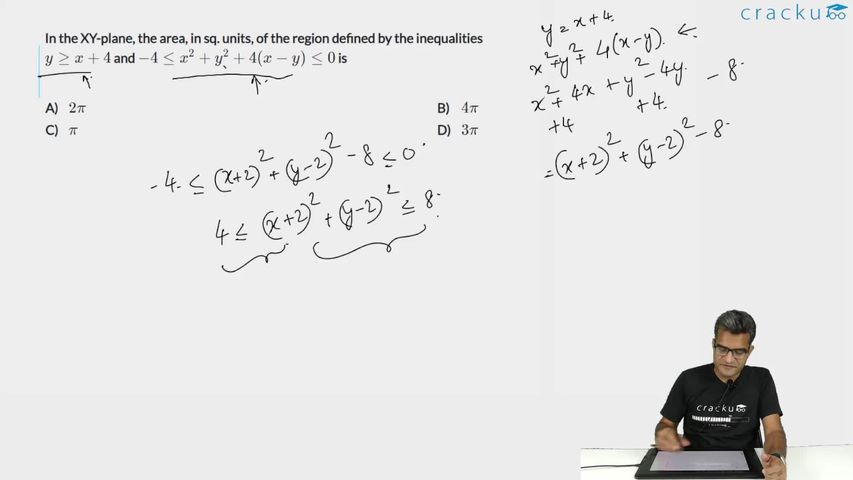
$$\ x^2\ +\ y^2\ +\ 4x\ -\ 4y\ +4\ \ge\ 0$$

$$\ x^2+\ 4x\ +4\ +\ y^2-\ 4y\ +4\ -4\ge\ 0$$

$$\left(x\ +\ 2\right)^2\ +\ \left(y\ -\ 2\right)^2\ \ge\ 4$$

The second inequality can be written as,

$$\ x^2\ +\ y^2\ +\ 4x\ -\ 4y\ \ \le\ \ 0$$

$$\ x^2+\ 4x\ +4\ +\ y^2-\ 4y\ +4\ -8\ \le\ \ 0$$

$$\left(x\ +\ 2\right)^2\ +\ \left(y\ -\ 2\right)^2\ \le\ \ 8$$

Representing all three inequalities in the graph, we get the graph as shown above, and we must calculate the intersection of all three inequalities,

We can see that the line passes through the centre of both circles (-2, 2), and the area obtained from the second inequality is the area between the two circles. So, the area of intersection of all three graphs is half of the area between both the circles as the line divides the circle in half, and we must only consider the area above the line as per the given inequality. We know that the area of the bigger circle is $$\sqrt{\ 8}$$ and the area of the smaller circle is $$\sqrt{\ 4}$$ from the equations of the circles as we know that equation of circle as $$\left(x\ -\ a\right)^2\ +\ \left(y\ -\ b\right)^2\ =\ \left(radius\right)^2$$ where (a,b) is the centre of the circle.

The area of intersection 

= $$\dfrac{1}{2}\left(Area\ of\ bigger\ circle\ -\ Area\ of\ smaller\ circle\right)$$

= $$\dfrac{1}{2}\left(\pi\ \left(\sqrt{\ 8}\right)^2\ -\pi\ \left(\sqrt{\ 4}\right)^2\right)$$

= $$\dfrac{1}{2}\left(8\pi\ \ -4\pi\right)$$

= $$\dfrac{1}{2}\left(4\pi\ \right)$$

= $$2\pi\ $$

Therefore, the correct answer is option A.