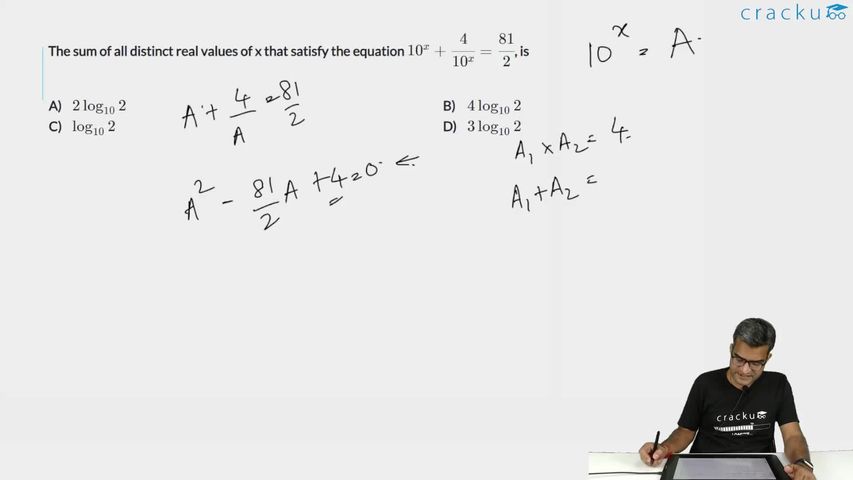
The sum of all distinct real values of x that satisfy the equation $$10^x + \cfrac{4}{10^x} = \cfrac{81}{2}$$, is

Taking $$10^x=a$$
we get $$a+\frac{4}{a}=\frac{81}{2}$$
This would give the quadratic equation: $$2a^2-81a+8=0$$

We want to find the sum of possible values of x, let the value of x be x1 and x2

these would correspond to log a1, and log a2

The sum of log a1 + log a2 would be log (a1 x a2)

From the quadratic equation we got above, we can see that the product of the possible values of a would-be 8/2 = 4

Threfore, the sum of values of x would be log (4) which would be $$2\ \log_{10}2$$

Therefore, Option A is the correct answer.