Please enter the following details
DIRECTIONS for the following three questions: Answer the questions on the basis of the information given below.
A city has two perfectly circular and concentric ring roads, the outer ring road (OR) being twice as long as the inner ring road (IR). There are also four (straight line) chord roads from E1, the east end point of OR to N2, the north end point of IR; from N1, the north end point of OR to W2, the west end point of IR; from W1, the west end point of OR, to S2, the south end point of IR; and from S1 the south end point of OR to E2, the east end point of IR. Traffic moves at a constant speed of $$30\pi$$ km/hr on the OR road, 20$$\pi$$ km/hr on the IR road, and 15$$\sqrt5$$ km/hr on all the chord roads.
The ratio of the sum of the lengths of all chord roads to the length of the outer ring road is
Let the radius of outer circle be 2R and the centre of both the circles be O.
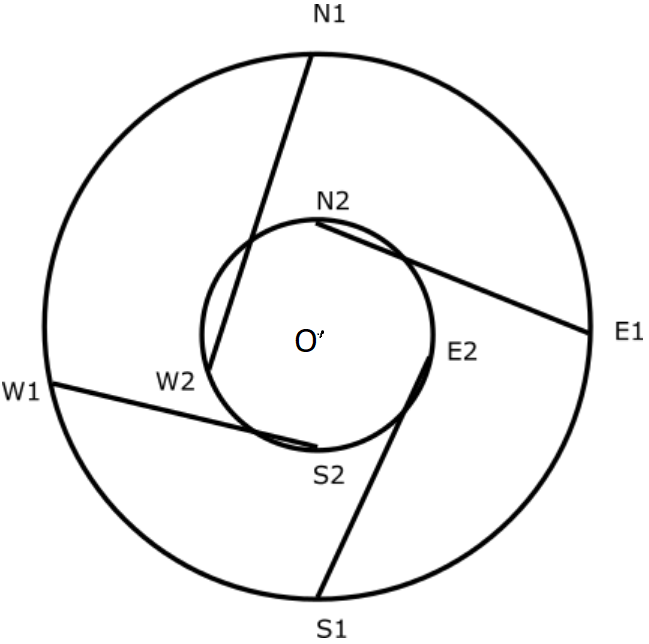
Triangle $$ON_2E_1$$and all the other 3 similar trianglesĀ form a right angle at the centre.Ā
Let the radius of the inner ring road be R
The radius of outer will be 2R as theĀ circumferenceĀ of the outer ring road is double that of the inner ring road.Ā
So, in triangleĀ $$ON_2E_1$$Ā using Pythagoras theorem the value of chords come out to be $$\sqrt5$$ * R so the total length of the chords 4 * $$\sqrt5$$ * R and circumference is equal to 2 *Pi*2R. The ratio gives option C.
Create a FREE account and get:
- All Quant CAT complete Formulas and shortcuts PDF
- 38+ CAT previous year papers with video solutions PDF
- 5000+ Topic-wise Previous year CAT Solved Questions for Free
