Sign in
Please select an account to continue using cracku.in
↓ →
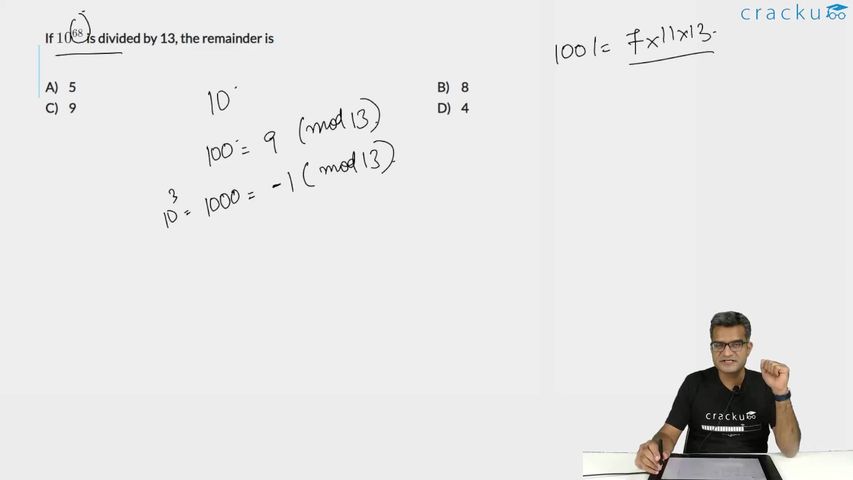
There are multiple ways of solving such questions involving remainders; one easy way is to look for a power of numerator that leaves a remainder of 1 or 01 when divided by the denominator.
In this instance, 1000, when divided by 13, leaves a remainder of -1
We can rewrite the numerator as $$\frac{10^{66}\times\ 100}{13}$$
The remainder would be $$\left[\frac{10^{66}}{13}\right]_R\times\ \left[\frac{100}{13}\right]_R$$
$$\left(-1\right)^{22}\times\ 9$$
9
Therefore, Option C is the correct answer.
Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get: