If $$(a + b\sqrt{3})^2 = 52 + 30\sqrt{3}$$, where a and b are natural numbers, then $$a + b$$ equals

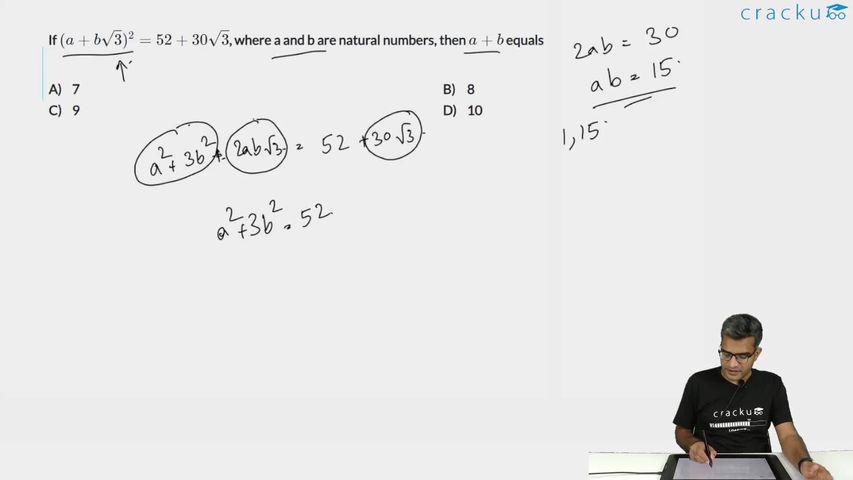
Opening the square on the left-hand side, we get $$a^2+3b^2+2ab\sqrt{\ 3}$$
Comparing the rational part on both side,s we get: $$a^2+3b^2=52$$
And comparing the irrational par,t we get: $$2ab\sqrt{\ 3}=30\sqrt{\ 3}$$

$$ab=15$$, Since we are given that a and b are natural numbers, the possible values of a and b are (1,15), (3, 5), (5, 3), or (15,1)

Putting these values in the first relation we got, we see that 15 squared would exceed the required value and would not be the case. 
We need not check if a=5, b=3 or a=3, b=5 since the answer would be the same. 

(a=5 and b=3 would satisfy it)

a+b = 5+3 = 8

Therefore, Option B is the correct answer.