A number is interesting if on adding the sum of the digits of the number and the product of the digits of the number, the result is equal to the number. What fraction of numbers between 10 and 100 (both 10 and 100 included) is interesting?

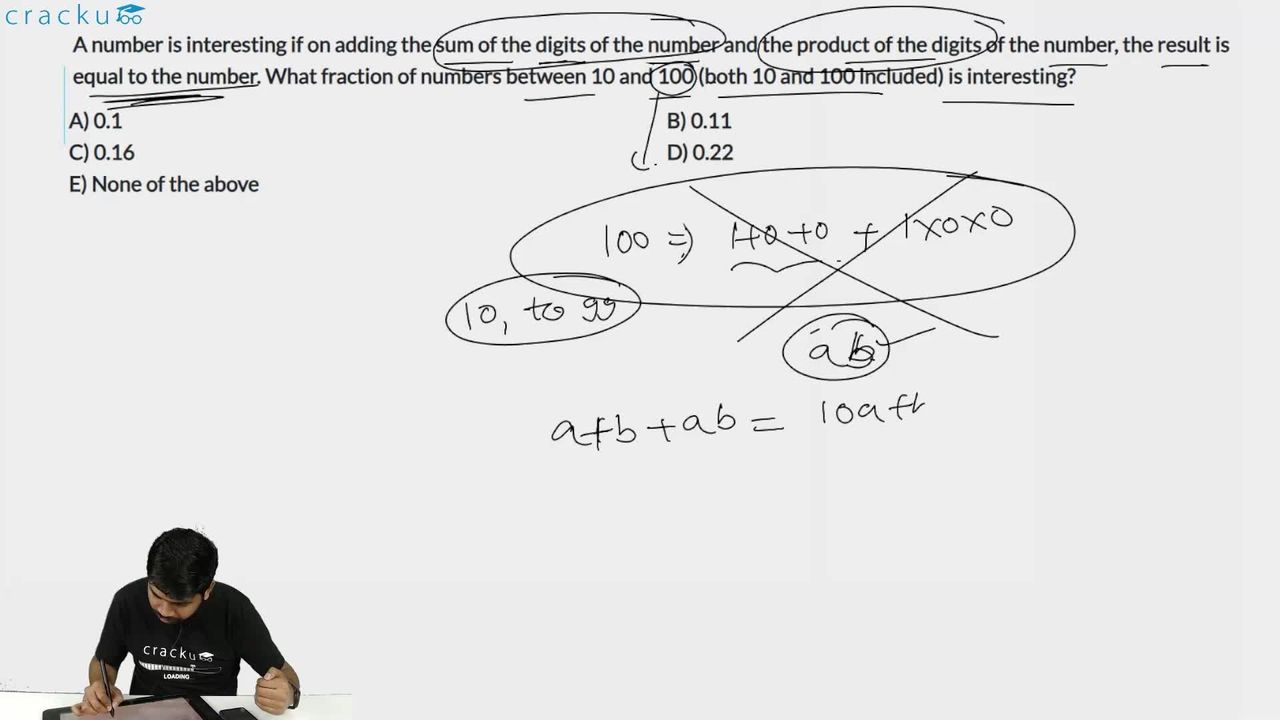
As the number is between 10 and 100 and 100 cannot be the number we are looking for, we can assume the number to be of two-digits.
Let the number be xy.
According to the question, for the number to be interesting
x + y + xy = 10x + y
On solving, we get 
xy = 9x
or, x (9 - y) = 0
x cannot be 0, because we need a number greater than or equal to 10.
So, 9 - y = 0
=> y = 9
For all the numbers whose unit digit is 9 will be an interesting number.
So, the numbers are 19, 29, 39, 49, .....99
There are 9 such numbers out of 91 total numbers between 10 and 100 including both.
Required fraction = $$\dfrac{9}{91}$$ = 0.0989
As this is not given in any of the options, the answer will be "none of the above".
Hence, option E is the correct answer.