Question 63
The figure below shows two right angled triangles ∆OAB and ∆OQP with right angles at vertex A and P, respectively, having the common vertex O, The lengths of some of the sides are indicated in the figure. (Note that the figure is not drawn to scale.) AB and OP are parallel.
What is ∠QOB?
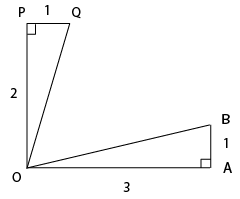
Solution
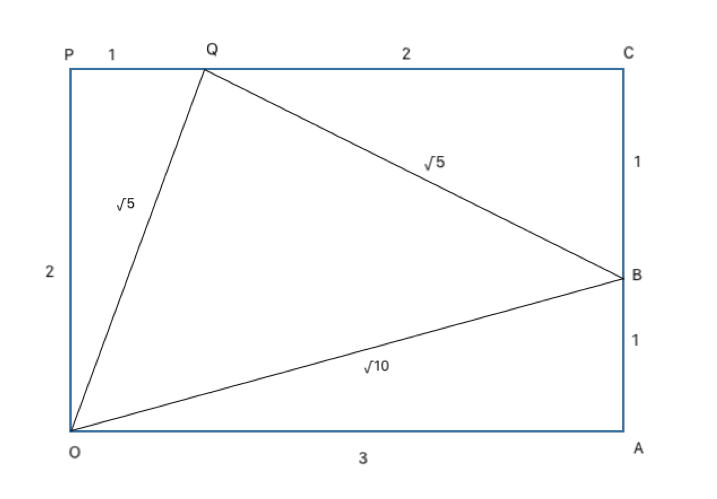
The figure can be redrawn as shown above.
$$\because $$ AB and OP are parallel, POAC is a rectangle.
In right $$\triangle$$QCB, QB = $$\sqrt{5}$$
$$\triangle$$QBO is an isosceles right angled triangle with $$\angle $$OQB = $$ 90°$$ (By Pythagoras theorem)
$$\therefore $$ $$\angle $$QOB = $$45°$$
Get AI Help
Video Solution
Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get:
- All Quant Formulas and shortcuts PDF
- 15 XAT previous papers with solutions PDF
- XAT Trial Classes for FREE
XAT Quant Questions | XAT Quantitative Ability
XAT Progressions and Series QuestionsXAT Inequalities QuestionsXAT Venn Diagrams QuestionsXAT Quadratic Equations QuestionsXAT Functions, Graphs and Statistics Questions
XAT DILR Questions | LRDI Questions For XAT
XAT Data Interpretation Basics QuestionsXAT Truth Lie Concept QuestionsXAT DI with connected data sets QuestionsXAT Quant Based DI QuestionsXAT Special Charts Questions
XAT Verbal Ability Questions | VARC Questions For XAT
Free XAT Quant Questions
XAT Data SufficiencyXAT Profit and LossXAT Venn DiagramsXAT GeometryXAT InequalitiesXAT Time, Speed and DistanceXAT Linear EquationsXAT Quadratic EquationsXAT Probability, CombinatoricsXAT Averages, Ratio and ProportionXAT Logarithms, Surds and IndicesXAT Progressions and SeriesXAT Functions, Graphs and StatisticsXAT Number Systems
