IPM Indore 2022 Question Paper

The sum of the coefficients of all the terms in the expansion of $$(5x - 9)^{4}$$ is __________.

$$\begin{bmatrix}1&0 & 0 \\ 0 &0&1\\ 0 & 1 & 0 \end{bmatrix}$$, then the absolute value of the determinant of $$(A9+A6+A3+A)$$ is ________.

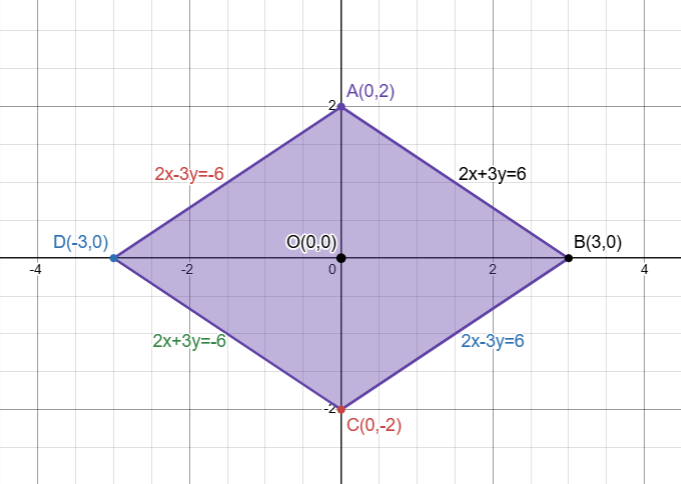
The area enclosed by $$2|x| + 3|y| \leq 6$$ is____________ sq. units

If $$\sin \alpha + \sin \beta = \frac{\sqrt{2}}{\sqrt{3}}$$ and $$\cos \alpha + \cos \beta = \frac{1}{\sqrt{3}}$$, then the value of $$(20 \cos (\frac{\alpha - \beta}{2}))^{2}$$ is_____

Mrs and Mr Sharma, and Mrs and Mr Ahuja along with four other persons are to be seated at a round table for dinner. If Mrs and Mr Sharma are to be seated next to each other, and Mrs and Mr Ahuja are not to be seated next to each other, then the total number of seating arrangements is _________.

Let P(X) denote power set of a set X. If A is the null set, then the number of elements in P(P(P(P(A)))) is

Let 50 distinct positive integers be chosen such that the highest among them is 100 , and the average of the largest 25 integers among them exceeds the average of the remaining integers by 50 . Then the maximum possible value of the sum of all the 50 integers is _________.

Aruna purchases a certain number of apples for INR 20 each and a certain number of mangoes for INR 25 each. If she sells all the apples at 10% profit and all the mangoes at 20% loss, overall she makes neither profit nor loss. Instead, if she sells all the apples at 20% loss and all the mangoes at 10% profit, overall she makes a loss of INR 150 . Then the number of apples purchased by Aruna is _________.

If $$\log_{(x^{2})}y + \log_{(y^{2})} x= 1$$ and $$y = x^{2} - 30$$, then the value of $$x^{2} + y^{2}$$ is _________

The numbers $$-16, 2^{x+3}, -2^{2x -1}-16, 2^{2x-1} + 16$$ are in an arithmetic progression. Then x equals _________.