What is the number of distinct triangles with integral valued sides and perimeter 14?

Let the sides be x, y and 14-(x+y)
x+y > 14-(x+y) => x+y > 7
x+14-x-y > y => y < 7
Similarly, x < 7
If x = 1, y = 7 (not possible)
So, if x = 2, y = 6
if x = 3, y = 5 if x = 4, y = 4, 5
The cases for x = 5 and 6 are already taken care of by y.
Number of possible cases = 4

Alternate solution::

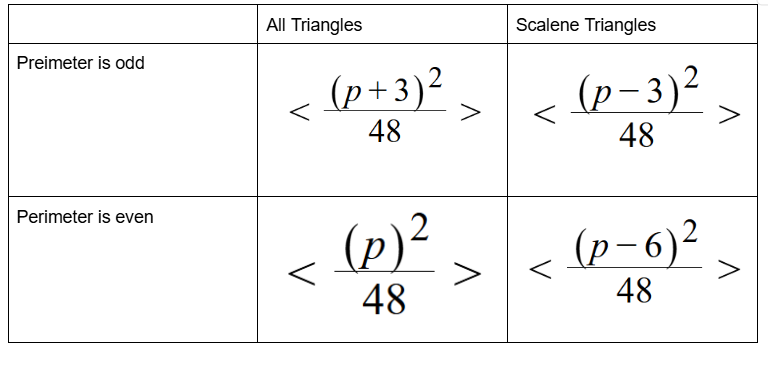
Here, the perimeter is even. So, the number of possible triangles is $$<\dfrac{p^2}{48}>$$ = $$<\dfrac{196}{48}>$$ = 4