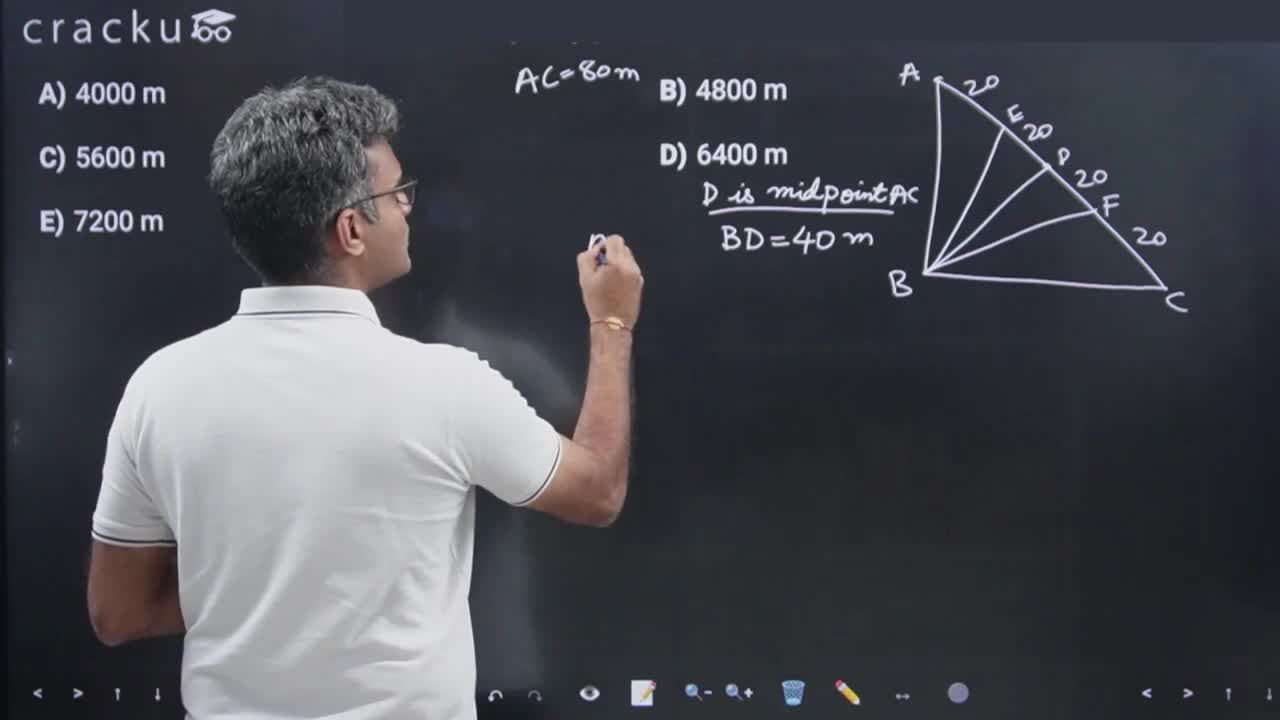
A city has a park shaped as a right angled triangle. The length of the longest side of this park is 80 m. The Mayor of the city wants to construct three paths from the corner point opposite to the longest side such that these three paths divide the longest side into four equal segments. Determine the sum of the squares of the lengths of the three paths.

To find : $$(BD)^2 + (BE)^2 + (BF)^2 = ?$$

AC = 80 m

AD = DE = EF = FC = 20

Let $$AB = a$$ and $$BC = b$$

In $$\triangle$$ ABC

$$(a)^2 + (b)^2 = (80)^2$$

Also, $$(BE) = 1/2 (AC) = 40 $$

=> $$BE = 40$$

Using Apollonius theorem in $$\triangle$$ ABE, as AD = DE

=> $$(AB)^2 + (BE)^2 = 2 [(BD)^2 + (AD)^2]$$

=> $$(BD)^2 + 20^2 = \frac{1}{2} (a^2 + 40^2)$$ --------Eqn(I)

Similarly, for $$\triangle$$ BEC, as EF = FC

=> $$(BE)^2 + (BC)^2 = 2 [(BF)^2 + (FC)^2]$$

=> $$(BF)^2 + 20^2 = \frac{1}{2} (b^2 + 40^2)$$ --------Eqn(II)

Adding eqns (I) & (II), we get :

=> $$(BD)^2 + (BF)^2 + 20^2 + 20^2$$ $$= \frac{1}{2} (a^2 + 40^2 + b^2 + 40^2)$$

=> $$(BD)^2 + (BF)^2 + 20^2 + 20^2$$ $$= \frac{1}{2} (80^2 + 40^2 + 40^2)$$

 => $$(BD)^2 + (BF)^2 = 4800 - 800 = 4000$$

$$\therefore$$ $$(BD)^2 + (BE)^2 + (BF)^2 = 4000 + 40^2$$

= $$4000 + 1600 = 5600$$