A square is inscribed in a circle of diameter 2a and another square is circumscribing circle. The difference between the areas of outer and inner squares is:

The given information can be shown in an image as follows,

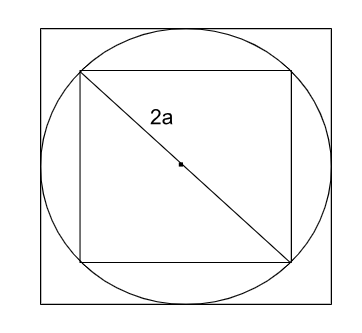
We can see that for the outer square, the length of the side is equal to the diameter of the circle. So, the side of the outer square is 2a, and the area of the outer square is $$2a\ \times\ 2a\ =\ 4a^2$$

We can see that for the inner square, the length of the diagonal is equal to the diameter of the circle. So, the area of the inner square is $$\dfrac{1}{2}\ \times\ 2a\ \times\ 2a\ =\ 2a^2$$.

So, the difference between the areas of the outer and the inner squares  = $$4a^2\ -\ 2a^2\ =\ 2a^2$$

Hence, the correct answer is option B.