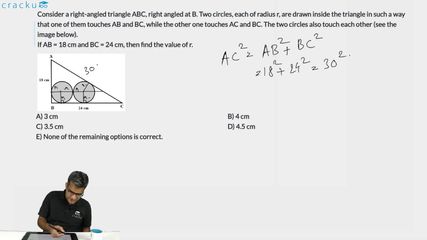
Consider a right-angled triangle ABC, right angled at B. Two circles, each of radius r, are drawn inside the triangle in such a way that one of them touches AB and BC, while the other one touches AC and BC. The two circles also touch each other (see the image below).
If AB = 18 cm and BC = 24 cm, then find the value of r.

Solution
It is given that ABC is a right-angled triangle, where $$\angle\ B\ =\ 90^{\circ\ }$$
Now, we can use Pythagoras theorem to calculate the value of AC, which is equal to $$\sqrt{\ 18^2+24^2}=\sqrt{\ 324+576}=\sqrt{\ 900}\ =\ 30$$ cm.

Here, we have drawn a common tangent MN, which is perpendicular to BC => Triangle MNC is a right-angled triangle.
It is also be concluded that traingle MNC is similar to traingle ABC
Hence, the ratio of the sides of triangle MNC is the same as the ratio of the sides of triangle ABC.
Thus, AB: BC: AC = MN: NC: MC = 18:24:30 = 3: 4: 5
Let the length of side MN be 3x => the length of NC = 4x, and the length of MC = 5x.
In triangle MNC, the circle inside it is the in circle of triangle MNC.
Thus, the in radius (r) of the circle in triangle MNC = (3x+4x-5x)/2 = x.
We know that BC = BN+NC, where BN is the diameter of the first circle, which is equal to 2r = 2x (since, r = x), and NC = 4x
=> 24 = 2x+4x
=> 6x = 24 => x = 4 cm.
Hence, the radius of the circle (x = r) is 4 cm.
The correct option is B
Video Solution
Click on the Email ☝️ to Watch the Video Solution
Create a FREE account and get:
- All Quant Formulas and shortcuts PDF
- 15 XAT previous papers with solutions PDF
- XAT Trial Classes for FREE
