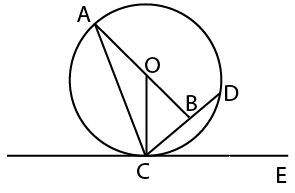
In the given figure, 0 is the centre of the circle and ∠DCE = 45°. If CD = $$10\sqrt2$$ cm, then what is the length (in cm) of AC . CB = BD

Given : CD = $$10\sqrt2$$ cm and BC = BD = $5\sqrt2$$

=> ∠OBC = 90°

Also, ∠DCE = 45°, => ∠OCB = 45°, => OB = BC ---------(i)

Now, in $$\triangle$$ OBC,

=> $$(OC)^2=(OB)^2+(BC)^2$$

=> $$(OC)^2=(5\sqrt2)^2+(5\sqrt2)^2$$

=> $$(OC)^2=50+50=100$$

=> $$OC=\sqrt{100}=10$$ cm

Similarly, in $$\triangle$$ ABC,

=> $$(AC)^2=(AB)^2+(BC)^2$$

=> $$(AC)^2=(10+5\sqrt2)^2+(5\sqrt2)^2$$

=> $$(AC)^2=100+50+100\sqrt2+50$$

=> $$(AC)^2=100(2+\sqrt2)=341.42$$

=> $$AC=\sqrt{341.42}=18.47\approx18.5$$ cm

=> Ans - (C)