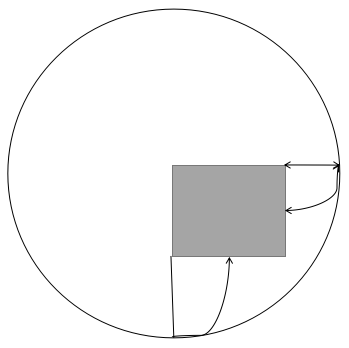
Rakhal is looking for a field where he can graze his cow. He finds a local farmer, Gopal, who agrees to rent his field to Rakhal for Rs. 1000 a year. Rakhal finds a post in the field and ties his cow to the post with a 25 feet rope. After some months, Gopal tells Rakhal that he will build a shed with four walls on the field with the post as one of the corner posts. The shed would be 15 feet by 10 feet. Rakhal agrees but he realizes that this arrangement would reduce the available area for grazing. What should be the modified rent to compensate for this loss of grazing area if Rakhal has to keep the cow tied to the same post with the same rope?
Solution
Original area for grazing = $$\pi \times (25)^2$$
= $$625 \pi$$ sq. feet
After the shed (15 $$\times$$ 10 feet) is built, quarter of the area will be reduced.
=> New area = $$\frac{3}{4} \pi \times (25)^2 + \frac{1}{4}[\pi (10)^2 + \pi (15)^2]$$
= $$\frac{1875 \pi}{4} + \frac{325 \pi}{4}$$
= $$\frac{2200 \pi}{4} = 550 \pi$$
Now, original area corresponds to Rs. 1,000
=> $$625 \pi \equiv 1000$$
$$\therefore 550 \pi \equiv 1000 \times \frac{550}{625}$$
= $$Rs. 880$$
Create a FREE account and get:
- All Quant Formulas and shortcuts PDF
- 15 XAT previous papers with solutions PDF
- XAT Trial Classes for FREE
