There are four machines in a factory. At exactly 8 pm, when the mechanic is about to leave the factory, he is informed that two of the four machines are not working properly. The mechanic is in a hurry, and decides that he will identify the two faulty machines before going home, and repair them next morning. It takes him twenty minutes to walk to the bus stop. The last bus leaves at 8:32 pm. If it takes six minutes to identify whether a machine is defective or not, and if he decides to check the machines at random, what is the probability that the mechanic will be able to catch the last bus?

As the mechanic has decided to check two machines, thus if he identifies either both defective machines or  both non defective, then the probability that he is able to catch the last bus is sum of the two. Thus, the possible outcomes are :

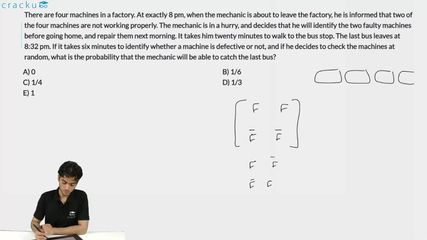
(i) : Both are defective machines, probability = $$(\frac{1}{2})(\frac{1}{3}) = \frac{1}{6}$$

(ii) : First is defective and second is non defective, probability = $$(\frac{1}{2})(\frac{2}{3}) = \frac{1}{3}$$

(iii) : First is non defective and second is defective, probability = $$(\frac{1}{2})(\frac{2}{3}) = \frac{1}{3}$$

(iv) : Both are non defective machines, probability = $$(\frac{1}{2})(\frac{1}{3}) = \frac{1}{6}$$

In the first and last cases, the mechanic would have identified the defective machines in time to catch the bus.

$$\therefore$$ Probability that he is able to catch the last bus = $$\frac{1}{6} + \frac{1}{6} = \frac{1}{3}$$

=> Ans - (D)