Question 20
A man is facing towards the east. He turns towards north and walks for 5 km, and then turns 270 degrees anticlockwise and walks for 12 km more. What is the minimum distance between his initial and final position?
Solution
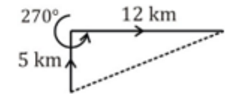
The man initially walked north for 5 km, then turned 270 degrees anticlockwise and walked towards east for 12 km.
Thus, minimum distance between his initial and final position
= $$\sqrt{(5)^2+(12)^2}$$
= $$\sqrt{25+144}=\sqrt{169}=13$$ km
=> Ans - (B)
Create a FREE account and get:
- Free SSC Study Material - 18000 Questions
- 230+ SSC previous papers with solutions PDF
- 100+ SSC Online Tests for Free
CAT Quant Questions | CAT Quantitative Ability
CAT Inequalities QuestionsCAT Averages, Ratio and Proportion QuestionsCAT Progressions and Series QuestionsCAT Percentages QuestionsCAT Profit, Loss and Interest Questions
CAT DILR Questions | LRDI Questions For CAT
CAT Data change over a period QuestionsCAT Arrangement QuestionsCAT Maxima-Minima QuestionsCAT Charts QuestionsCAT Selection With Condition Questions
