Two posts are 4 m apart. Both posts are on same side of a tree. If the angles of depressions of these posts when observed from the top of the tree are $$45^\circ$$ and $$60^\circ$$ respectively, then what is the height of the tree?

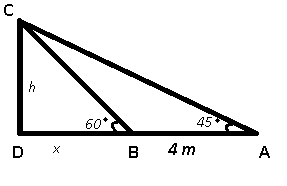
Given : CD is the tree and AB = 4 m

To find : Height of tree = $$h$$ = ?

Solution : In $$\triangle$$ ACD,

=> $$tan(45^\circ)=\frac{CD}{AD}$$

=> $$1=\frac{h}{x+4}$$

=> $$h=x+4$$ -------------(i)

Again, in $$\triangle$$ BCD,

=> $$tan(60^\circ)=\frac{CD}{DB}$$

=> $$\sqrt{3}=\frac{h}{x}$$

=> $$h=x\sqrt{3}$$

=> $$h=(h-4)\sqrt3$$     [Using (i)]

=> $$h=h\sqrt3-4\sqrt3$$

=> $$h(\sqrt3-1)=4\sqrt3$$

=> $$h=\frac{4\sqrt3}{\sqrt3-1}$$

Rationalizing the denominator, we get :

=> $$h=\frac{4\sqrt3}{\sqrt3-1}\times\frac{(\sqrt3+1)}{(\sqrt3+1)}$$

=> $$h=\frac{4\sqrt3(\sqrt3+1)}{(3-1)}$$

=> $$h=2\sqrt3(\sqrt3+1)$$

=> Ans - (C)