You can find the important CAT Number System questions from previous papers, complete with detailed video explanations, on this page. The number system is a crucial part of the CAT quantitative section. There are numerous tricks, shortcuts, and formulas that can help you solve these questions quickly. These solving tips are explained in the video solutions by CAT experts and IIM alumni. This is your one-stop resource for practicing the CAT Number Systems concept.
Additionally, take free CAT mock tests to understand the exam pattern and get a good idea of the types of questions asked. You can download the CAT number systems questions PDF with detailed solutions and practice to excel in the quant section. The best part is that you can download the questions PDF for free without signing up. Click the link below to download all the number system questions from previous CAT papers in PDF format.
Question 1
Zahir and Raman are at the entrance of a dark cave. To enter this cave, they need to open a number lock. Raman sees a note on a rock: “ ... chest of pure diamonds kept for the smart one ... number has six digits ... second last digit is 2, third last is 4 ... divisible by all prime numbers less than 15 ...”. Excited, Zahir and Raman seek your help: which of these can be the first digit of the six-digit number that will help them open the lock?
correct answer:-5
Question 2
In a six-digit number, the sixth, that is, the rightmost, digit is the sum of the first three digits, the fifth digit is the sum of first two digits, the third digit is equal to the first digit, the second digit is twice the first digit and the fourth digit is the sum of fifth and sixth digits. Then, the largest possible value of the fourth digit is
correct answer:-7
Question 3
How many 4-digit numbers, each greater than 1000 and each having all four digits distinct, are there with 7 coming before 3?
correct answer:-315
Question 4
The mean of all 4-digit even natural numbers of the form 'aabb',where $$a>0$$, is
correct answer:-4
Question 5
How many pairs(a, b) of positive integers are there such that $$a\leq b$$ and $$ab=4^{2017}$$ ?
correct answer:-1
Question 6
How many 3-digit numbers are there, for which the product of their digits is more than 2 but less than 7?
correct answer:-21
Question 7
Let m and n be natural numbers such that n is even and $$0.2<\frac{m}{20},\frac{n}{m},\frac{n}{11}<0.5$$. Then $$m-2n$$ equals
correct answer:-2
Question 8
What is the remainder if $$19^{20} - 20^{19}$$ is divided by 7?
correct answer:-1
Question 9
If a, b and c are positive integers such that ab = 432, bc = 96 and c < 9, then the smallest possible value of a + b + c is
correct answer:-4
Question 10
When expressed in a decimal form, which of the following numbers will be non - terminating as well as non-repeating?
correct answer:-3
Question 11
The product of two positive numbers is 616. If the ratio of the difference of their cubes to the cube of their difference is 157:3, then the sum of the two numbers is
correct answer:-3
Question 12
For all possible integers n satisfying $$2.25\leq2+2^{n+2}\leq202$$, then the number of integer values of $$3+3^{n+1}$$ is:
correct answer:-7
Question 13
Wilma, Xavier, Yaska and Zakir are four young friends, who have a passion for integers. One day, each of them selects one integer and writes it on a wall. The writing on the wall shows that Xavier and Zakir picked positive integers, Yaska picked a negative one, while Wilma’s integer is either negative, zero or positive. If their integers are denoted by the first letters of their respective names, the following is true:
$$W^{4}+X^{3}+Y^{2}+Z\leq4$$
$$X^{3}+Z\geq2$$
$$W^{4}+Y^{2}\leq2$$
$$Y^{2}+Z\geq3$$
Given the above, which of these can $$W^{2}+X^{2}+Y^{2}+Z^{2}$$ possibly evaluate to?
correct answer:-4
Question 14
Let N, x and y be positive integers such that $$N=x+y,2<x<10$$ and $$14<y<23$$. If $$N>25$$, then how many distinct values are possible for N?
correct answer:-6
Question 15
How many integers in the set {100, 101, 102, ..., 999} have at least one digit repeated?
correct answer:-252
Question 16
How many of the integers 1, 2, … , 120, are divisible by none of 2, 5 and 7?
correct answer:-2
Question 17
An encryption system operates as follows:
Step 1. Fix a number k $$(k \leq 26)$$.
Step 2. For each word, swap the first k letters from the front with the last k letters from the end in reverse order. If a word contains less than 2k letters, write the entire word in reverse order.
Step 3. Replace each letter by a letter k spaces ahead in the alphabet. If you cross Z in the process to move k steps ahead, start again from A.
Example: k = 2: zebra --> arbez --> ctdgb.
If the word “flight” becomes “znmorl” after encryption, then the value of k:
correct answer:-5
Question 18
For a 4-digit number, the sum of its digits in the thousands, hundreds and tens places is 14, the sum of its digits in the hundreds, tens and units places is 15, and the tens place digit is 4 more than the units place digit. Then the highest possible 4-digit number satisfying the above conditions is
correct answer:-4195
Question 19
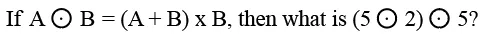
correct answer:-3
Question 20
A supplier receives orders from 5 different buyers. Each buyer places their order only on a Monday. The first buyer places the order after every 2 weeks, the second buyer, after every 6 weeks, the third buyer, after every 8 weeks, the fourth buyer, every 4 weeks, and the fifth buyer, after every 3 weeks. It is known that on January 1st, which was a Monday, each of these five buyers placed an order with the supplier.
On how many occasions, in the same year, will these buyers place their orders together excluding the order placed on January 1st?
correct answer:-3
![Top Important CAT Number System Questions [Download PDF] Top Important CAT Number System Questions [Download PDF]](/media/CAT_Quant_-_Number_System_-__Questions_With_Detailed_Solutions_.webp)











![Top Important CAT Number System Questions [Download PDF] Top Important CAT Number System Questions [Download PDF]](/media/cache/0b/2e/0b2e5f8e097aff36ce62f1f10f334480.webp)
![Most Important CAT Averages Questions [PDF] Most Important CAT Averages Questions [PDF]](/media/cache/54/e8/54e80dac6301b8d404ec410ed272b262.webp)













