The chord of a circle is 10 cm in length and at a distance of $$\frac{10}{\sqrt3}$$cm from the centre. Calculate the angle subtended by the chord at the centre of a circle.

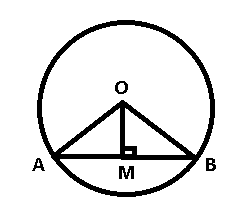
Given : AB = 10 cm and OM = $$\frac{10}{\sqrt3}$$ cm

To find : $$\angle$$ AOB = $$\theta$$ = ?

Solution : Perpendicular from the centre to the chord bisects the chord, => MB = $$\frac{10}{2}=5$$ cm

Also, $$\angle$$ AOB = $$2\angle$$ MOB

=> $$\angle$$ MOB = $$\frac{\theta}{2}$$ ------------(i)

Now, in $$\triangle$$ OMB

=> $$tan(\angle MOB)=\frac{MB}{OM}$$

=> $$tan(\frac{\theta}{2})=5\div\frac{10}{\sqrt3}$$

=> $$tan(\frac{\theta}{2})=5\times\frac{\sqrt3}{10}$$

=> $$tan(\frac{\theta}{2})=\frac{\sqrt3}{2}$$

=> $$\frac{\theta}{2}=tan^{-1}(\frac{\sqrt3}{2})$$

=> $$\theta=2 \tan^{-1}(\frac{\sqrt{3}}{2})$$

=> Ans - (A)