D and E are points on side AB and AC of ΔABC. DE is parallel to BC. If AD:DB = 2:3, what is the ratio of area of ΔADE and area of quadrilateral BDEC?

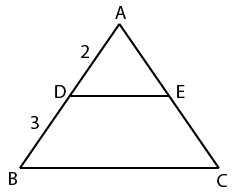
ADE & ABC are similar, let area of $$\triangle$$ ABC = y, that of ADE = x

For similar triangles

Ratio of sides = $$\sqrt{ \text(ratio of areas)}$$

AB/AD = $$\sqrt{ \frac{area.ABC}{area.ADE}}$$

5/2 = $$\sqrt{ \frac{y}{x}}$$

25/4 = $$\frac{y}{x}$$

y = 25x/4

 area of quadrilateral BDEC = ABC - ADE = y - x = 25x/4 - x = 21x/4

$$\frac{ADE}{BDEC} = \frac{x}{21x/4} = \frac{4x}{21x} = \frac{4}{21}$$

So the answer is option A.