Question 31
.png)
In the given figure, two identical circles of radius 4 cm touch each other. A and B are the centres of the two circles. If RQ is a tangent to the circle, then what is the length (in cm) of RQ?
Solution
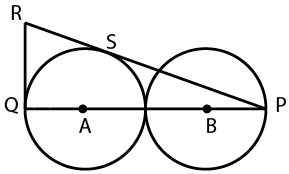
We can say RQ = RS =xÂ
Now PQ =16Â
Also if you consider P to be an external point with respect to circle with center A
Let circles touch each other at M
so we get (PM)(PQ) =PS^2
we get 16(8) =PS^2
so PS =$$8\sqrt{\ 2}$$
Now In triangle PQR
we get $$RQ^2+PQ^2=PR^2$$
We get :
$$x^2+256=\left(x+8\sqrt{\ 2}\right)^2$$
we get $$16\sqrt{\ 2}x\ =128$$
x=$$4\sqrt{\ 2}$$
so RQ =$$4\sqrt{\ 2}$$
Create a FREE account and get:
- Free SSC Study Material - 18000 Questions
- 230+ SSC previous papers with solutions PDF
- 100+ SSC Online Tests for Free
CAT Quant Questions | CAT Quantitative Ability
CAT Time Speed Distance QuestionsCAT Averages QuestionsCAT Time, Distance and Work QuestionsCAT Data Sufficiency QuestionsCAT Linear Equations Questions
CAT DILR Questions | LRDI Questions For CAT
CAT Puzzles QuestionsCAT Miscellaneous LR QuestionsCAT DI with connected data sets QuestionsCAT DI Miscellaneous QuestionsCAT Data change over a period Questions
