Question 136

A chord AB of a circle C1 of radius (√3 + 1) cm touches a circle C which is concentric to C . If the radius of C is (√3 -1) cm., the length of AB is :

Solution

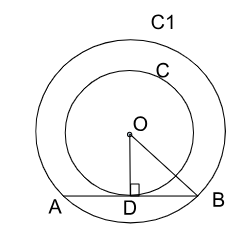
OB = $$(\sqrt{3} + 1)$$ cm

OA = $$(\sqrt{3} - 1)$$ cm

In right $$\triangle$$ OAB

=> $$(AB)^2 = (OB)^2 - (OA)^2$$

=> $$(AB)^2 = (\sqrt{3} + 1)^2 - (\sqrt{3} - 1)^2$$

=> $$(AB)^2 = (4 + 2\sqrt{3}) - (4 - 2\sqrt{3})$$

=> $$(AB)^2 = 4\sqrt{3}$$

=> $$AB = \sqrt{4\sqrt{3}}$$

=> $$AB = 2\sqrt[4]{3}$$

Create a FREE account and get: