Question 133
The distance between centers of two circles of radii 3 cm and 8 cm is 13 cm. If the points of contact of a direct common tangent to the circles are P and Q, the length of the line segment PQ is:
Solution
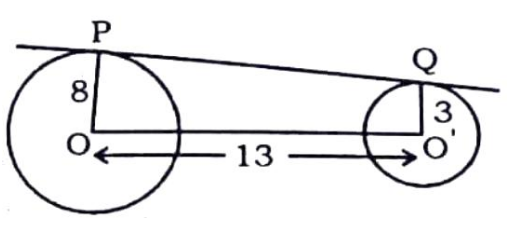
Two circles having radii $$r_1$$ and $$r_2$$ and distance between them $$d$$
Length of direct common tangent PQ = $$\sqrt{d^2-(r_2-r_1)^2}$$
= $$\sqrt{(13)^2-(8-3)^2}$$
= $$\sqrt{169-25}=\sqrt{144}=12$$ cm
=> Ans - (C)
Get AI Help
Create a FREE account and get:
- Free SSC Study Material - 18000 Questions
- 230+ SSC previous papers with solutions PDF
- 100+ SSC Online Tests for Free
