Question 108
If $$x$$ and $$y$$ are positive real numbers satisfying $$x + y = 52$$ , then the minimum possible value of $$91(1 + \frac{1}{x})(1 + \frac{1}{y})$$ is:
Solution
Lets expand the given equation.
$$91\left(1+\frac{1}{x}+\frac{1}{y}+\frac{1}{xy}\right)$$
$$91\left(1+\frac{x+y}{xy}+\frac{1}{xy}\right)$$
x+y is constant, 91 is constant.
To get the minimum value we have to minimixe the xy term.
That happens when both are equal.
So x=26, y=26.
$$91\left(\left(1+\frac{1}{26}\right)\left(1+\frac{1}{26}\right)\right)$$
On simiplifing we get $$\frac{5103}{52}$$
Get AI Help
Video Solution
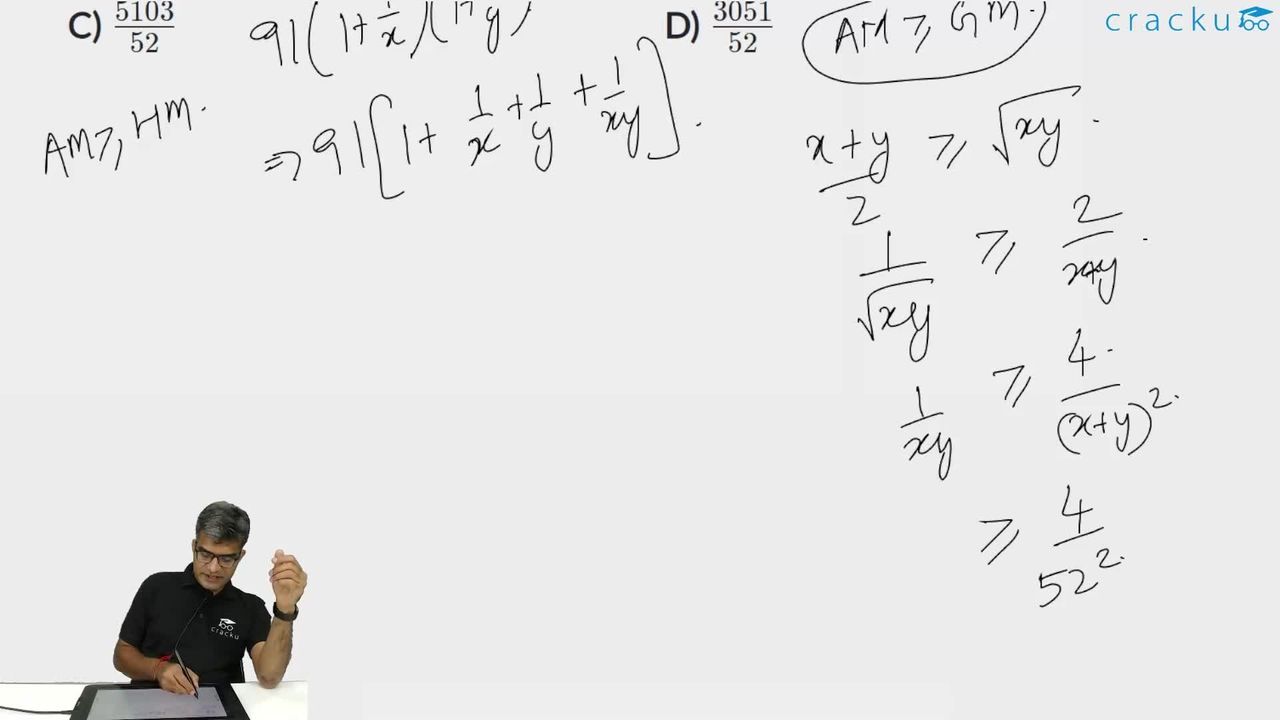
Click on the Email ☝️ to Watch the Video Solution
