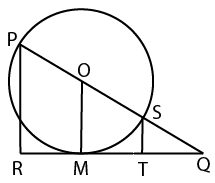
In the given figure. PR and ST are perpendicular to tangent QR. PQ passes through centre 0 of the circle whose diameter is 10 cm. If PR = 9 cm. then what is the length (in cm) of ST? 

Given : PR = 9 cm and radius OM = PO = OS = 5 cm

To find : ST = $$x$$ = ?

Solution : Let SQ = $$y$$ cm

In $$\triangle$$ PRQ and $$\triangle$$ OMQ

=> $$\angle$$ PRQ = $$\angle$$ OMQ = $$90^\circ$$

and $$\angle$$ PQR = $$\angle$$ OQM    (common angle)

=> $$\triangle$$ PRQ $$\sim$$ $$\triangle$$ OMQ 

=> $$\frac{PR}{PQ}=\frac{OM}{OQ}$$

=> $$\frac{9}{10+y}=\frac{5}{5+y}$$

=> $$45+9y=50+5y$$

=> $$9y-5y=50-45$$

=> $$y=\frac{5}{4}$$ -------------(i)

Similarly, in $$\triangle$$ PRQ and $$\triangle$$ STQ

=> $$\frac{PR}{PQ}=\frac{ST}{SQ}$$

=> $$\frac{9}{10+y}=\frac{x}{y}$$

=> $$9y=10x+xy$$

=> $$9\times\frac{5}{4}=x(10+\frac{5}{4})$$

=> $$\frac{45}{4}=x\frac{45}{4}$$

=> $$x=ST=1$$ cm

=> Ans - (A)