In the given figure. PQ = 30. RS = 24 and OM = 12. then what is the value of ON?
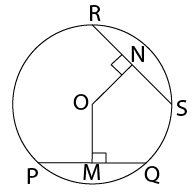
Solution

Given : PQ = 30, RS = 24 and OM = 12
To find : ON = ?
Solution : A perpendicular from the centre of a circle to the chord bisects it.
=> MQ = $$\frac{3}{2}=15$$ and NS = 12
In $$\triangle$$ OMQ,
=> $$(OQ)^2=(OM)^2+(MQ)^2$$
=> $$(OQ)^2=(12)^2+(15)^2$$
=> $$(OQ)^2=144+225=369$$
Also, OQ = OS = radii of circle
Similarly, in $$\triangle$$ ONS,
=> $$(ON)^2=(OS)^2-(NS)^2$$
=> $$(ON)^2=369-(12)^2$$
=> $$(ON)^2=369-144=225$$
=> $$ON=\sqrt{225}=15$$
=> Ans - (C)
Create a FREE account and get:
- Free SSC Study Material - 18000 Questions
- 230+ SSC previous papers with solutions PDF
- 100+ SSC Online Tests for Free

