Question 71

From an external point P, a tangent PQ is drawn to a circle, with the centre O, touching the circle at Q. If the distance of P from the centre is 13 cm and length of the tangent PQ is 12 cm, then the radius of the circle is:

Solution

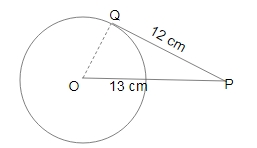
$$\triangle$$ OPQ is a right angle triangle because $$\angle Q = 90\degree$$,

By Pythagoras,

$$(OQ)^2 + (PQ)^2 = (OP)^2$$

$$(OQ)^2 = (13)^2 - (12)^2$$

$$(OQ)^2 = 169 - 144$$

$$(OQ)^2 = 25$$

$$OQ = 5$$

$$\therefore$$ The radius of the circle is 5 cm.

Create a FREE account and get:

Free SSC Study Material - 18000 Questions
230+ SSC previous papers with solutions PDF
100+ SSC Online Tests for Free

CAT Quant Questions | CAT Quantitative Ability

CAT Profit And Loss Questions CAT Arithmetic Questions CAT Coordinate Geometry Questions CAT Quadratic Equations Questions CAT Probability, Combinatorics Questions

CAT DILR Questions | LRDI Questions For CAT

CAT Selection With Condition Questions CAT DI Miscellaneous Questions CAT Coins and Weights Questions CAT Venn Diagrams Questions CAT Charts Questions

CAT Verbal Ability Questions | VARC Questions For CAT

CAT Grammar and Sentence Correction Questions CAT Para Summary Questions CAT Verbal Ability Questions CAT Reading Comprehension Questions CAT Miscellaneous Questions

Follow us on

Boost your Prep!

Download App